This question was posted here on StackExchange.
Let $A$ be a commutative ring and $B,C$ be two commutative $A$-algebras. Consider the pushout square of ring homomorphism $\require{AMScd}$ \begin{CD} A@>\beta>>B\\ @V\gamma VV@VVV\\ C@>>>B\otimes_AC \end{CD} In this answer it is proved that the corresponding commutative square of spectrum $\DeclareMathOperator\Spec{Spec}$ \begin{CD}\tag 1 \Spec(B\otimes_AC)@>>>\Spec(B)\\ @VVV@VV\Spec(\beta)V\\ \Spec(C)@>>\Spec(\gamma)>\Spec(A) \end{CD} is a pullback in the category of schemes.
I'm looking for sufficient conditions on $A\to C$ which make $(1)$ a pullback square in category of sets for every $A\to B$.
Two such conditions are:
- if $C=A/\mathfrak a$ for some ideal $\mathfrak a$ of $A$;
- if $C=S^{-1}A$ for some mutliplicative system $S$ of $A$.
Note that in both case, $A\to C$ is an epimorphism of commutative rings; so my question is:
If $A\to C$ is an epimorphism of commutative rings, then $(1)$ is a pullback of sets for every ring homomorphisms $A\to B$?
My try.
Let $\beta:A\to B$, $\gamma:A\to C$ and $\tau:A\to B\otimes_AC$ and consider the pushout square of commutative rings above.
If $\gamma:A\to C$ is a ring epimorphism, then the right-handed $B\to B\otimes_AC$ is a ring epimorphism as well.
Moreover, it's know that the functions $\Spec(B\otimes_AC)\to\Spec(B)$ and $\Spec(C)\to\Spec(A)$ are injective.
By [Atiyah & MacDonald - ex. 25 pag. 48] we have
$$\operatorname{Im}\Spec(\tau)=\operatorname{Im}\Spec(\beta)\cap\operatorname{Im}\Spec(\gamma)$$
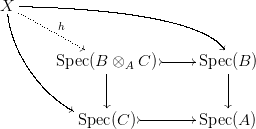
This proves that for every set $X$ there exists a function $h:X\to\Spec(B\otimes_AC)$ and the injectivity of $\Spec(C)\to\Spec(A)$ implies that the left-handed triangle commutes. I've troubles in showing that the upper triangle commutes as well.
