The diagram below indicates 2 ways to use Fenchel-Nielsen coordinates to parameterize the Teichmüller space of conformal structures on the 4-holed sphere with totally-geodesic boundary, corresponding to the two pants decompositions, as indicated by this diagram:
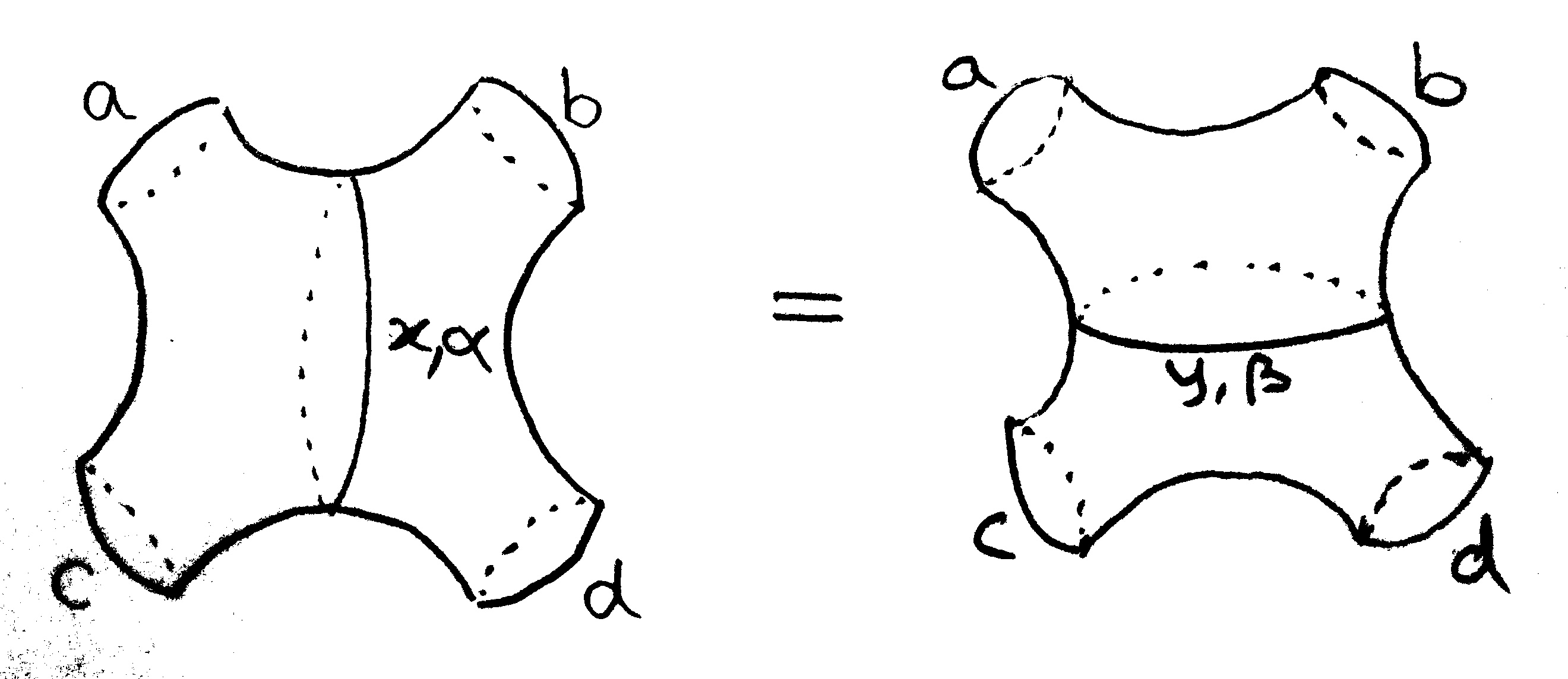
Here $a,b,c,d,x,y$ are lengths of the indicated geodesics in a hyperbolic instance of the conformal structure, and $\alpha,\beta$ are the twisting parameters for the gluing.
What is the coordinate transformation implied by these decompositions? That is, what is the formula for $y$ and $\beta$ as a function of $a,b,c,d,x,\alpha$?
