Let $\mathcal{G}$ be a Lie groupoid. The target map $t:\mathcal{G}_1\rightarrow \mathcal{G}_0$ is a principal $\mathcal{G}$ bundle.
This article Orbifolds as Stacks? by Eugene Lerman calls (in page $11$) this particular principal bundle to be the unit principal $\mathcal{G}$ bundle. So, for a Lie groupoid $\mathcal{G}$, this $\mathcal{G}$ bundle is a special element in $B\mathcal{G}$.
Let $\mathcal{G}$ and $\mathcal{H}$ be Lie groupoids. For $B\mathcal{G}$, I have a special element $t:\mathcal{G}_1\rightarrow \mathcal{G}_0$. For $B\mathcal{H}$, I have a special element $t:\mathcal{H}_1\rightarrow \mathcal{H}_0$. We can ask the follwing question.
Are "the unit elemnts" preserved by an arbitrary map of stacks $F:B\mathcal{G}\rightarrow B\mathcal{H}$ i.e., do we always have $F(t:\mathcal{G}_1\rightarrow \mathcal{G}_0)=t:\mathcal{H}_1\rightarrow \mathcal{H}_0$ for any map of stacks $F:B\mathcal{G}\rightarrow B\mathcal{H}$.
This question does not make sense. As $F$ preserves fibers, $F(t:\mathcal{G}_1\rightarrow \mathcal{G}_0)$ would be a principal $\mathcal{H}$ bunle of the form $Q\rightarrow \mathcal{G}_0$. So, $F(t:\mathcal{G}_1\rightarrow \mathcal{G}_0)$ which is of the form $Q\rightarrow \mathcal{G}_0$ can not be the principal $\mathcal{H}$ bundle $t:\mathcal{H}_1\rightarrow \mathcal{H}_0$.
We have principal $\mathcal{H}$ bundles $Q\rightarrow \mathcal{G}_0$ and $\mathcal{H}_1\rightarrow \mathcal{H}_0$. As base spaces are different there is no way (in general) to compare these two principal $\mathcal{H}$ bundles.
Suppose we are in a situation where $B\mathcal{G}\rightarrow B\mathcal{H}$ is coming from a morphism of Lie groupoids $\mathcal{G}\rightarrow \mathcal{H}$. Realising that there is an obvious map between base spaces of these two bundles namely $\phi:\mathcal{G}_0\rightarrow \mathcal{H}_0$ makes the question of looking for some relation between $Q\rightarrow \mathcal{G}_0$ and $\mathcal{H}_1\rightarrow \mathcal{H}_0$ more specific. The question would then be,
Is $Q\rightarrow \mathcal{G}_0$ same the pull back of $t:\mathcal{H}_1\rightarrow \mathcal{H}_0$ along $\phi:\mathcal{G}_0\rightarrow \mathcal{H}_0$.
In a very special case when this morphism of Lie groupoids $\phi:\mathcal{G}\rightarrow \mathcal{H}$ is a Lie groupoid extension i.e., when $\phi:\mathcal{G}_0\rightarrow \mathcal{H}_0$ is an identity map (and some thing extra), we can ask
Is $Q\rightarrow \mathcal{G}_0$ same thing as $t:\mathcal{H}_1\rightarrow \mathcal{H}_0=\mathcal{G}_0$.
I could not see why this is true from definition of map of stacks but I feel this should be the case. Any comments are welcome.
Edit : A stack (over the category of manifolds $\text{Man}$) for me is a category $\mathcal{D}$ along with a functor $\mathcal{D}\rightarrow \text{Man}$ such that it is a category fibered in groupoids and some extra conditions. By an element of stack I mean an element (object) in the category $\mathcal{D}$.
Consider the special case when this map of stacks $B\mathcal{G}\rightarrow B\mathcal{H}$ is coming from a morphism of Lie groupoids $\mathcal{G}\rightarrow \mathcal{H}$. I will recall how one gets $B\mathcal{G}\rightarrow B\mathcal{H}$ from $\mathcal{G}\rightarrow \mathcal{H}$.
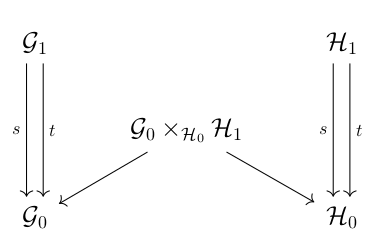
We first construct a $\mathcal{G}-\mathcal{H}$ bibundle given $\phi:\mathcal{G}\rightarrow \mathcal{H}$. We consider principal $\mathcal{H}$ bundle $t:\mathcal{H}_1\rightarrow \mathcal{H}_0$, pull it back along $\phi:\mathcal{G}_0\rightarrow \mathcal{H}_0$ to get a principal $\mathcal{H}$ bundle, now with the base $\mathcal{G}_0$ which is precisely $\mathcal{G}_0\times_{\mathcal{H}_0}\mathcal{H}_1\rightarrow \mathcal{H}_0$. The manifold $\mathcal{G}_0\times_{\mathcal{H}_0}\mathcal{H}_1$ has an action of $\mathcal{G}$ on it, given by $g.(x,h)=(t(g),\phi(g)h)$. With this action, it becomes a $\mathcal{G}-\mathcal{H}$ bibundle.
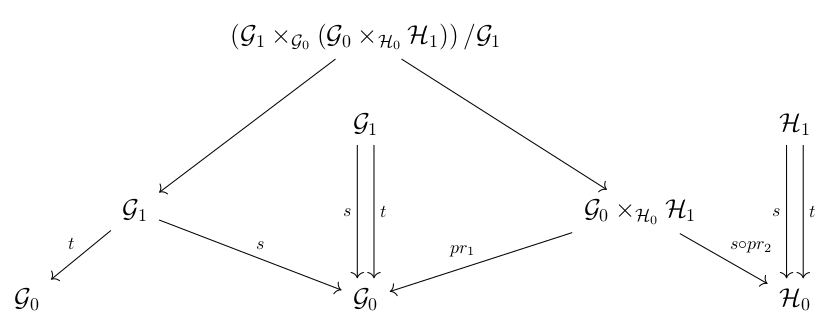
This $\mathcal{G}-\mathcal{H}$ bibundle gives morphism of stacks $B\mathcal{G}\rightarrow B\mathcal{H}$ the map is given by composition of bibundle. The object $\mathcal{G}_1\rightarrow \mathcal{G}_0$ is mapped to $\left(\mathcal{G}_1\times_{\mathcal{G}_0}(\mathcal{G}_0\times_{\mathcal{H}_0}\mathcal{H}_1)\right)/\mathcal{G}_1\rightarrow \mathcal{G}_0$ which comes from following diagram
We have $\left(\mathcal{G}_1\times_{\mathcal{G}_0}(\mathcal{G}_0\times_{\mathcal{H}_0}\mathcal{H}_1)\right)/\mathcal{G}_1= \mathcal{G}_0\times_{\mathcal{H}_0}\mathcal{H}_1$.
So, the principal bundle is $\mathcal{G}_0\times_{\mathcal{H}_0}\mathcal{H}_1\rightarrow \mathcal{G}_0$.
If $\mathcal{G}\rightarrow \mathcal{H}$ is a Lie groupoid extension, then we have $\mathcal{H}_0=\mathcal{G}_0$ and $\mathcal{G}_0\times_{\mathcal{H}_0}\mathcal{H}_1=\mathcal{H}_1$. So, $\mathcal{G}_0\times_{\mathcal{H}_0}\mathcal{H}_1\rightarrow \mathcal{G}_0$ is just the target map $t:\mathcal{H}_1\rightarrow \mathcal{H}_0$.
So, if $F:B\mathcal{G}\rightarrow B\mathcal{H}$ is given by a morphism of Lie groupoids $\mathcal{G}\rightarrow \mathcal{H}$ which is a Lie groupoid extension, then $F(t:\mathcal{G}_1\rightarrow M)=t:\mathcal{H}_1\rightarrow M$.
Otherwise, it does not make sense to ask if $F$ takes unit principal bundle of $\mathcal{G}$ to unit principal bundle of $\mathcal{H}$.