Re: 'is it customary'. Yes, this is the customary term, and I don't know any reasonable alternative to using the eccentricity function. The term (with exactly this definition) already occurs on page 35 of the slim yet influential textbook
[H1969] Frank Harary, Graph Theory, Addison Wesley Publishing Company, First Edition, 1969
Re "who first used it to define graph centers": needless to say, this cannot be known, but again it is at least as old as [H1969, p. 35], where one reads
"The *eccentricity $e(v)$ of a point $v$ in a connected graph $G$ is $\max d(u,v)$ for all $u$ in $G$. The radius $r(G)$ is the minimum eccentricity of the points. Note that the maximum eccentricity is the diameter. A point $v$ is a central point if $e(G)=r(G)$, and the center of $G$ is the set of all central points.
[bold emphasis added]
Re "what was the motiviation/justification for using eccentricity in that definition": this can only be guessed or answered by authors like Harary themselves. That said, a reasonable just-so-story is this: consider the definition 'center=set of vertices whose eccentricity equals the graph's radius', and then note that in the most paradigmatic shape having a 'center', i.e, the humble circle, the definition makes perfect sense: for any point $p$ in a circle define its 'eccentricity' to be the Euclidean length of the segment from $p$ through the circle's centre $c$ to the circle's circumference; then the one-element set $\{c\}$ equals the set of all points of the circle whose eccentricity equals the radius. The story goes that the early graph theorists were wont to scrawl with pens on paper, and often these shapes were circular, and this gave them ideas.
In that regard, let me close with a warning: the 'center' thus defined does not alway look quite so intuitively 'central'; e.g. in the graph represented by
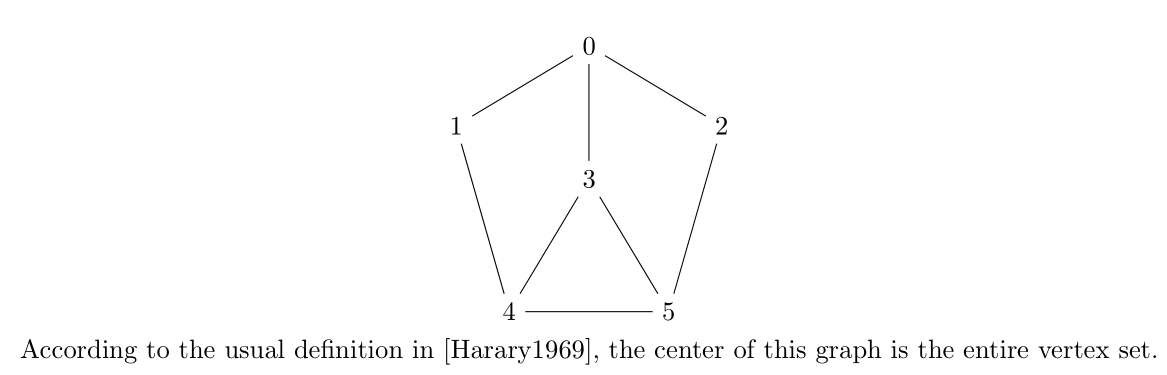 [graph and caption by present author]
[graph and caption by present author]
each and every vertex is central (with eccentricity 2), and yet the automorphism group of the graph acts on the vertex set with a whopping four distinct orbits $\{0\}$, $\{3\}$, $\{1,2\}$, $\{4,5\}$. (In other words, whereas all vertices are being lumped together into the same 'eccentricity-class', there exist quite distinct-looking vertices, and there are four types of vertices.)

