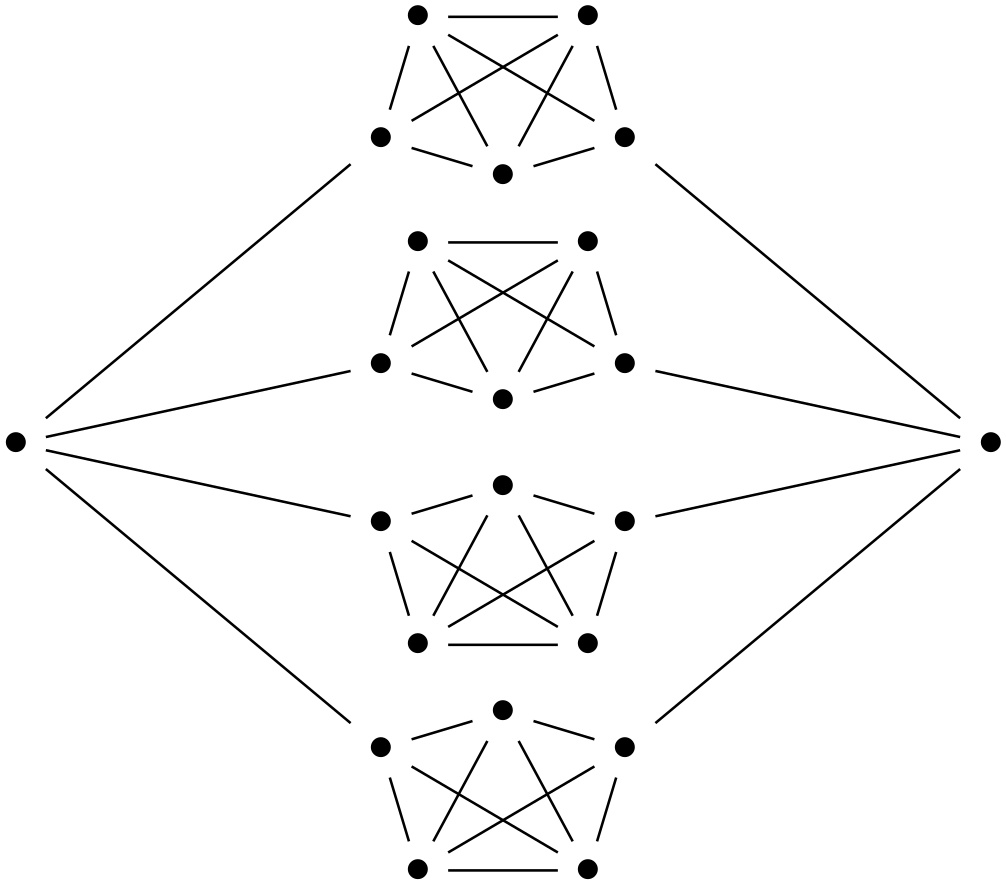
In this paper (Construction 2.6 p860) the authors have built examples of connected $k$-regular graph without Hamiltonian path, but with a cut-vertex (i.e. it is not $2$-connected).
Question: Is there a $2$-connected $k$-regular graph without Hamiltonian path?
Remark: every $2$-connected $k$-regular graph with at most $3k+3$ vertices admits a Hamiltonian cycle or is one of the two exceptions, which admit a Hamiltonian path.