Let $C$ be an infinite, separable and connected metric space. If $C$ becomes totally disconnected when one of its points $p\in C$ is removed, does every closed ball of $C$ with positive radius and center $p$ always contain an infinite connected subset?
-
1$\begingroup$ I have a question: if C becomes totally disconnected when the point p is removed, does it necessarily mean that every connected component of C has to include p? Thx for clarifying. $\endgroup$– Rachid AtmaiCommented Aug 18, 2010 at 23:15
-
$\begingroup$ @alephomega: I believe the answer is yes. For if $B\subset C$ and contains more then one point, but $p\notin B$, then when removing $p$ we still have $B\subset C\setminus\{p\}$, and $B$ is still connected, which is a contradiction to the totally disconnectedness of $C\setminus \{p\}$. $\endgroup$– Dror AtariahCommented Aug 19, 2010 at 8:07
2 Answers
Here I construct an example which proves the answer is NO.
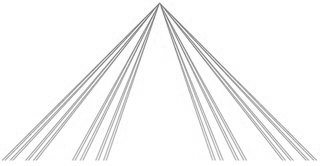
Take the KK fan:
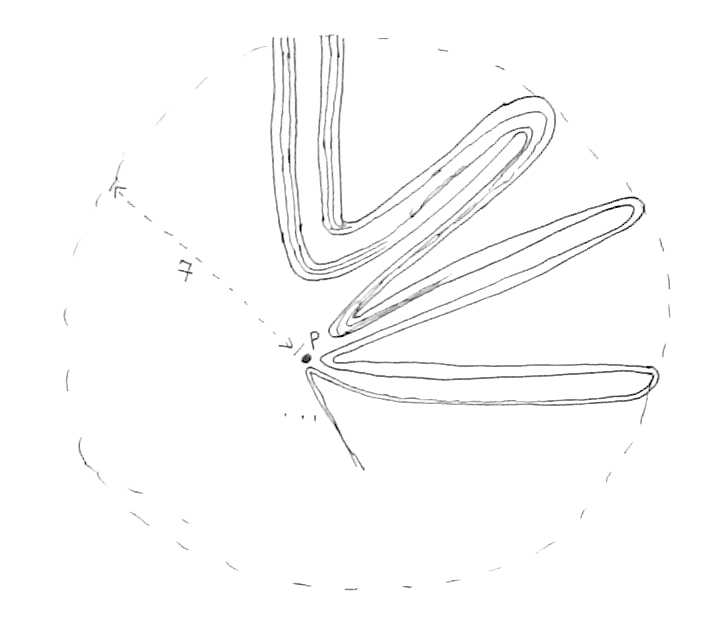
Remove its dispersion point at the top. Now you have a Cantor set of lines of rationals/irrationals that cannot be separated horizontally. Stretch this into a "Cantor-like tube" and weave it closer and closer to a point $p$ in the plane while shrinking its diameter and making sure that every loop goes a distance of $7$ away from $p$.
Remove $p$ and you have a hereditarily disconnected space ($\simeq$ KK fan minus its vertex). If $A$ is nonempty and clopen in $X$ then $A$ must snake around the tube forever, so it limits to $p$ and thus $p\in A$. Therefore $X$ is connected. The ball of radius $1$ around $p$ is hereditarily disconnected.
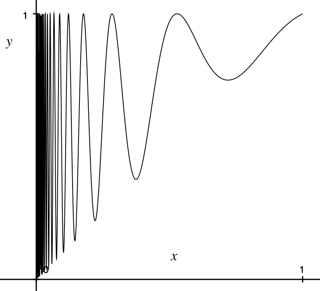
EDIT: I am basically taking the space which consists of the curve below, and the origin $p=(0,0)$ (so $\{0\}\times (0,1]$ is not included). The difference is that instead of weaving a line, I am weaving this "Cantor tube" while shrinking its diameter. In the first case if I remove $p$ then I get something $\simeq [0,\infty)$, whereas in the second case I get something $\simeq$ my Cantor tube.
-
$\begingroup$ Be patient. As for me I like it very much but have not digested it yet. $\endgroup$ Commented Apr 30, 2016 at 19:56
-
$\begingroup$ Why removing $p$ gives $\simeq$ KK minus vertex? $\endgroup$ Commented Apr 30, 2016 at 20:01
-
$\begingroup$ Aren't you creating new converging sequences by weaving closer and closer to $p$? The points involved will become more and more closer to each other... $\endgroup$ Commented Apr 30, 2016 at 20:08
-
$\begingroup$ The only convergent sequences will converge to $p$. The tube does not limit to any point of itself. Maybe it is not clear in the picture, but the loops will stop before they get to, say, the 9:00 position. $\endgroup$ Commented Apr 30, 2016 at 20:11
-
$\begingroup$ But there are infinitely many of them, and their points which were apart in KK get closer and closer to each other $\endgroup$ Commented Apr 30, 2016 at 20:30
I don't know if this answers your question precisely but here's an interesting example. First, let's start with the following:
The Knaster-Kuratowski Fan
Let $K$ be the Knaster-Kuratowski fan, also called Cantor's teepee. The space $K$ is defined as follows. Let $C$ be the Cantor set. Let $Q \subset C$ be the set of endpoints of the deleted middle-third intervals. Let $P=C \setminus Q$. We also let $p=(1/2,1/2) \in \mathbb R^2$. Now for each $x \in C$, we let $L_x$ be the line joining $p$ and $x$. Now our space $K$ is the union over all $x \in C$ of the sets
- $\{ (x,y) \in L_x : y\in \mathbb Q \}$, if $x\in Q$, and
- $\{ (x,y) \in L_x : y\notin \mathbb Q \}$, if $x \in P$.
The space $K$ is connected but $K\setminus \{p\}$ is totally disconnected. Also $K$ is punctiform, that is it contains no compact connected T2 subspace.
A related property of $K$
Let $f$ be a continuous function from $K$ to $K$. Let $U$ be some closed connected open set about a point $x \neq p$. Then there exists a closed connected open set $V$ about $p$, which can be written down as the set of all $(x,y)$ with $1/2 - \epsilon < y < 1/2$ such that $f(V) \subset U$ since $f$ is continuous. Then $V$ is necessarily homeomorphic to the space $K$.