Does there exist a more general (than Malliavin or Itô) "Stochastic calculus" defined on $L^1$ space, or some Orlicz space between $L^2$ and $L^1$?
For examples: are there:
- Ito Isometry(-types) of results for L1 processes
Does there exist a more general (than Malliavin or Itô) "Stochastic calculus" defined on $L^1$ space, or some Orlicz space between $L^2$ and $L^1$?
For examples: are there:
Note that even for usual Ito calculus wrt to Brownian motion, there is a variation of Ito isometry for processes with infinite variance see. https://nualart.ku.edu/StochasticCalculus.pdf
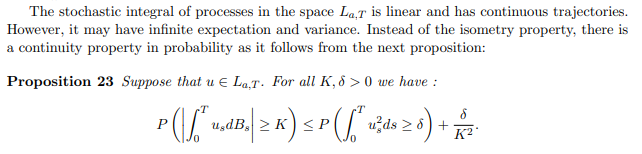
Now in terms of a calculus in terms of general processes that are not in $L^{2}$, it gets hard because we lose the Hilbert-space structure and thus nice things such as orthonormality/projections (eg. "Stochastic Calculus with Respect to Gaussian Processes"). Even in Rough-paths, one needs some regularity to do calculus.
One reference I found online for processes with infinite variance is "On generalized Malliavin calculus".