1
$\begingroup$

Let $R$ be a commutative ring with identity. D.G. Northcott's, Finite Free Resolutions, has:
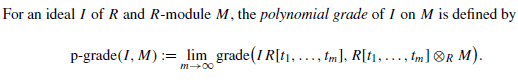
enter image description here

and in Theorem 16 of Chapter 5 proves that: $p.grade(I,M) = p.grade(P,M)$ for some prime ideal $P$ containing $I$ .

Question. Let $I$ be a finitely generated ideal. Can one claim that there is a finitely generated prime ideal $P$ containing $I$ such that $p.grade(I,M) = p.grade(P,M)$?

Thank you

Improve this question
$\endgroup$
2
  • $\begingroup$ Why do you need P finitely generated ?, what for ? The aim of Northcott's theory of grade is to avoid finiteness of ideal-generators (the ground ring is any commutative ring, not necessary Noetherian ). $\endgroup$
    – Al-Amrani
    Commented Nov 24, 2015 at 9:56
  • $\begingroup$ yes the ring is not necessary Noetherian, in the Question too. but it can have finitely generated ideal $\endgroup$
    – user 1
    Commented Nov 24, 2015 at 20:04

1 Answer 1

Reset to default
1
$\begingroup$

No, for an ideal may be finitely generated without being contained in any finitely generated prime ideal. For example, the zero ideal in a non-noetherian zero-dimensional local ring has this property.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .