differential equation of conics

Question

Function $y(x)$ on the plane defines a conic (strictly speaking, at most second order algebraic curve) if and only if $\frac{d^3}{dx^3} (y'')^{-2/3}=0$. What is intuition behind this? How may I see without calculations that this equation is symmetric in $x$, $y$, or, say, that it is affine invariant? Quick calculation comes from considering Wronskian of 6 functions $1,x,y,x^2,y^2,xy$ which vanishes iff $(x,y)$ lie on a conic. But it is not quite satisfactory explanation.

Answer 1

I didn't see this question when it originally appeared, but an edit today brought it to the front page of "new questions" where I saw it. Back around 2005 or 2006 I came across this differential equation for conics (see this 26 April 2008 sci.math post, where I gave a formal-algebraic derivation of it), and ever since then I've been collecting copies of papers that discuss this equation when I happen to come across them. Although I have not made a systematic literature search on this topic, the references below (listed in chronological order) should give anyone interested in doing such a literature search a good starting point. In this regard, Mukhopadhyay [5] seems like the best place to begin.

[1] Georges Henri Halphen (1844-1889), Sur l'équation différentielle des coniques [On the differential equation of the conics], Bulletin de la Société Mathématique de France 7 (1879), 83-85. MR1503806; JFM 11.0250.02

Halphen states that, in a paper in Volume 4 of BSMF (neither the paper's title nor a page number is given), he had indicated a way of obtaining a 5th degree differential equation whose solutions give all conics. The present paper gives another way to obtain the same equation. Beginning with $y = ax + b + \sqrt{Ax^2 + 2Bx + C},$ we differentiate twice to obtain $y'' = (AC – B^2)(Ax^2 + 2Bx + C)^{-\frac{3}{2}}.$ Thus, $(y'')^{-\frac{2}{3}}$ is an arbitrary 2nd degree polynomial, and hence we get $\left[(y'')^{-\frac{2}{3}}\right]''' = 0.$ When expanded, this becomes $9(y'')^2y''''' – 45y''y'''y'''' + 40(y''')^3 = 0.$ Halphen also remarks that $\left[(y'')^{-\frac{2}{3}}\right]'' = 0$ is a differential equation for all parabolas. The remaining 60% to 70% of Halpen's paper is devoted to showing how one can solve the differential equation for conics by certain substitutions and the use of standard methods.

[2] Thomas Muir (1844-1934), The differential equation of a conic, Philosophical Magazine (5) 21 #129 (February 1886), 143-145. JFM 18.0244.01

(first sentence of the paper) THE differential equation of a conic, $$9\left(\frac{d^{2}y}{dx^2}\right)^{2}\frac{d^{5}y}{dx^5} \;\; - \;\; 45\frac{d^{2}y}{dx^2}\frac{d^{3}y}{dx^3}\frac{d^{4}y}{dx^4} \;\; + \;\; 40\left(\frac{d^{3}y}{dx^3}\right)^3 \;\; = \;\; 0,$$ which Boole attributes to Monge, and to which Sylvester in this week's 'Nature' (vol. xxxiii. p. 224) so fully directs attention, can be readily obtained as follows.

(last paragraph of the paper) In the 'Proceedings of the Edinb. Math. Soc.' 1884-85, pp. 95-100, Professor Chrystal has a note on "A Method for obtaining the Differential Equation of an Algebraical Curve." In the case of the cubic he gets for the non-zero side of the equation a determinant of the 6th order which he says can be simplified further. I find that when this simplification is made, and $y_{2}, \, y_{3}, \, \ldots$ are expressed in terms of $\alpha, \, \beta, \, \ldots,$ the result agrees perfectly with the above*.

footnote * states: The differential equation of the cubic appears to have been first given by Mr. Samuel Roberts in the 'Educational Times.' The question and solution are reprinted in 'Math. from Educ. Times,' x. pp. 47, 48. The date of the first publication of the question, which unfortunately can in no case be got with certainty from the Reprint, should probably be 1868.---T. M. (January 18).

[3] Allan Joseph Champneys Cunningham (1842-1928), Depression of differential equations, Messenger of Mathematics 17 (1888), 118-145. JFM 19.0296.01

another link to the paper

The differential equation of conics is dealt with on pp. 140-145.

(from p. 140) As an example of these principles, the equation--- $$9{y''}^{2}y^v \; - \; 45y''y'''y^{iv} \; + \; 40{y'''}^{3} \; = \; 0,$$ which is the well known differential equation of a conic may be taken. It wants [means: It does not have] $x, \, y, \, y',$ and has multiple homogeneity, so may be depressed five orders in all, the result being an algebraic equation.

(end of the paper) Hence the 5 depressions required in all may be effected in any of the following orders $[ \cdots]$ But this procedure is of course not so easy as that before shewn in abstract in Tables VIII. and IX., especially in the ascending steps, the reversal of Step II. not being so easy as in the former procedure. It is proposed to show the detail in a further paper.

[4] Allan Joseph Champneys Cunningham (1842-1928)], Depression of differential equations, Messenger of Mathematics 18 (1889), 122-127. JFM 20.0330.02

another link to the paper

(first 2 sentences of the paper) THIS paper is only a supplement to the paper on Depression of Differential Equations in pp. 118 to 145 of Vol. XVII. It contains the detail of three additional modes of solution (by depression) of the differential equation of a conic, $$9{y''}^{2}y^v \; - \; 45y''y'''y^{iv} \; + \; 40{y'''}^{3} \; = \; 0,$$ not quite so simple as those given at pp. 140 to 144 of above.

[5] Asutosh Mukhopadhyay [Mookerjee] (1864-1924), The geometric interpretation of Monge's differential equation to all conics, Journal of the Asiatic Society of Bengal 58 (Part 2) #2 (1889), 181-185.

(first 1.5 pages of the paper) Before proceeding to give the true geometric interpretation of Monge's differential equation to all conics, which I have recently discovered, and which it is the object of this paper to announce and establish, a brief survey of the past history and present position of the problem may not be wholly unprofitable. In the first place, then, we remark that the differential equation of all conics was, more than three-quarters of a century ago, first discovered by the illustrious French mathematician Gaspard Monge, and published by him in 1810.† {footnote † states: Sur les Equations différentielles des Courbes du Second Degré. (Bulletin de la Soc. Philom. Paris, 1810, pp. 87-88; Corresp. sur l'E'cole Polytech. (Hachette) Paris, 1809-13, t. ii, pp. 51-54).} It should be remembered that, in his paper, Monge does not furnish us with any clue to the method by which, from the integral equation of the conic, he derived the differential equation which now appropriately bears his name: neither is there any attempt at a geometric interpretation; it is simply stated that the differential equation to all conics of the second order as obtained by the elimination of the constants from the equation $$Ay^2 + 2Bxy + Cx^2 + 2Dy + 2Ex + 1 = 0$$ is $$9q^{2}t \; – \; 45qrs \; + \; 40r^3 \; = \; 0,$$ where, as usual, $$p = \frac{dy}{dx}, \;\;\; q = \frac{d^{2}y}{dx^2}, \;\;\; r = \frac{d^{3}y}{dx^3}, \;\;\; s = \frac{d^{4}y}{dx^4}, \;\;\; t = \frac{d^{5}y}{dx^5};$$ and this statement is followed by a verification that the differential equation of all circles $$(1 + p^2)r \; = \; 3pq^2$$ leads, on differentiation, to the differential equation of all conics.

After Monge's paper, we come to the following statement made by the late Dr. Boole:‡ {footnote ‡ is: Differential Equations, Fourth Edition, pp. 19-20.} "Monge has deduced the general differential equation of lines [= curves] of the second order, expressed by the algebraic equation $$ax^2 + bxy + cy^2 + ex + fy = 1.$$ It is $$9\left(\frac{d^{2}y}{dx^2}\right)^{2}\frac{d^{5}y}{dx^5} \;\; - \;\; 45\frac{d^{2}y}{dx^2}\frac{d^{3}y}{dx^3}\frac{d^{4}y}{dx^4} \;\; + \;\; 40\left(\frac{d^{3}y}{dx^3}\right)^3 \;\; = \;\; 0$$ But, here our powers of geometrical interpretation fail, and results such as this can scarcely be otherwise useful than as a registry of integrable forms."

The subject seems to have attracted the notice of English mathematicians from the above statement of Boole, and, during the thirty years which have elapsed since these remarks were first made, there appear to have been two attempts to interpret geometrically Monge's differential equation to all conics. The first of these propositions, by Lieut.-Col. Cunningham, is that the eccentricity of the osculating conic of a given conic is constant all round the latter.* {footnote * is: Quarterly Journal of Mathematics (1877), vol. xiv, pp. 226-229.} The second propositioin, by Prof. Sylvester, is that the differential equation of a conic is satisfied at the sextactic points of any given curve.† {footnote † is: American Journal of Mathematics (1886), vol. ix, pp. 18-19.} I have elsewhere considered in detail both these propositions, and I have fully set forth my reasons for holding that neither of them is the true geometric interpretation of Monge's defferential [sic] equation to all conics.‡ {footnote ‡ is: Journal, A. S. B. (1887), vol. lvi, part ii, pp. 134-145; P. A. S. B. (1887), pp. 185-186; P. A. S. B. (1888), pp. 74-86.}

[6] Edwin Bailey Elliott (1851-1937), Note on the differential equation of a conic, Messenger of Mathematics 19 (1890), 5-7. JFM 21.0332.01

another link to the paper

(first paragraph of the paper) It is a surprisingly prevalent idea that the integration of $${y''}^{2}y^v \; - \; 5y''y'''y^{iv} \; + \; \frac{40}{9}{y'''}^{3} \; = \;0,$$ the differential equation of a conic, is a matter of considerable difficulty. The recent papers† {footnote † is: Vol. XVII., pp. 118-145, Vol. XVIII., pp. 122-127.} of Colonel Cunningham on the depression of differential equations must have done much to dispel the notion; but his processes of depression and integration of the equation occupy, as he says, some considerable space when written at length. It may be worth while then to show how simple the matter really is by writing down two variations of a perfectly direct and elementary method of performing the integration.

[7] Francis Sowerby Macaulay (1862-1937) [journal editor], [Solution to Problem 143], Examination Questions and Problems [column], Mathematical Gazette 1 #10 (February 1897), 94-95.

JSTOR link to the item

Original statement of the problem in Mathematical Gazette 1 #9 (October 1896), p. 67. No attribution is given except an indication that the problem appeared on a recent Oxford University examination.

Statement of problem: Prove that the result of eliminating the constants in the general equation of a conic by differentiation is $$\frac{d^3}{dx^3}\left\{\left(\frac{d^{2}y}{dx^2}\right)^{-\frac{2}{3}}\right\} = 0.$$

Published solution: Let the equation of the conic be $$ax^2 + 2hxy + by^2 + 2gx + 2fy + c \; = \; 0.$$ Solving for $y,$ we have $$y \; = \; Dx + E \; \pm \; (Ax^2 + 2Bx + C)^{\frac{1}{2}},$$ where $A,$ $B,$ $C,$ $D,$ $E$ are constants. Differentiating, $$\frac{dy}{dx} \; = \; D \; \pm \; \frac{Ax + B}{(Ax^2 + 2Bx + C)^{\frac{1}{2}}};$$ and $$\frac{d^{2}y}{dx^2} \; = \; \pm \frac{A(Ax^2 + 2Bx + C) \; - \; (Ax + B)^2}{(Ax^2 + 2Bx + C)^{\frac{3}{2}}} \;\; = \;\; \pm \frac{AC \; - B^2}{(Ax^2 + 2Bx + C)^{\frac{3}{2}}};$$ $$\therefore \;\; \left(\frac{d^{2}y}{dx^2}\right)^{-\frac{2}{3}} \;\; = \;\; (AC \; – \; B^2)^{-\frac{2}{3}}(Ax^2 + 2Bx + C);$$ $$\therefore \;\;\frac{d^3}{dx^3}\left\{\left(\frac{d^{2}y}{dx^2}\right)^{-\frac{2}{3}}\right\} = 0.$$

[8] François Olivier Raoul Perrin (1841-1910), Sur quelques consequences géométriques de l'équation différentielle des coniques [On some geometrical consequences of the differential equation of the conics], Bulletin de la Société Mathématique de France 31 (1903), 54-64. MR1504438; JFM 34.0633.02

This paper studies the differential equation $9(y'')^2y''''' – 45y''y'''y'''' + 40(y''')^3 = 0,$ which has all planar conic curves for its solutions.

[9] François Olivier Raoul Perrin (1841-1910), Sur les intégrales de l'équation différentielle des coniques et leur interprétation géométrique [On the integrals of the differential equation of the conics and their geometric interpretation], Bulletin de la Société Mathématique de France 31 (1903), 275-285. MR1504459; JFM 34.0633.03

This paper continues the investigation begun in Perrin's previous paper.

Answer 2

I don't know what you mean by 'without calculation'. I don't think you'll get a simpler explanation than simply solving the equation: If $$ \frac{d^3\bigl((y'')^{-2/3}\bigr)}{dx^3} = 0, $$ then $$ y''(x) = (ax^2 +2bx + c)^{-3/2} $$ for some constants $a$, $b$, and $c$, so, integrating twice (assuming $ac-b^2\not=0$), you get $$ y(x) = ex + f + \frac{(ax^2 +2bx + c)^{1/2}}{(ac-b^2)} $$ for some constants $e$ and $f$, which gives $$ (ac-b^2)^2(y-ex-f)^2 - (ax^2 +2bx + c) = 0, $$ which is the general equation of a conic. (I'm sure you can handle the degenerate cases.)

Obviously, this is reversible, so what more is there to know?

Finally, you should be aware that the expression $\frac{d^3\bigl((y'')^{-2/3}\bigr)}{dx^3}$ is not, itself, an affine invariant, let alone a projective invariant: It's not even homogeneous of degree $0$ in $y$. The true projective invariant is the symmetric cubic form $$ C = (y'')^{2/3}\left(\frac{d^3\bigl((y'')^{-2/3}\bigr)}{dx^3}\right)\,dx^3. $$

Answer 3

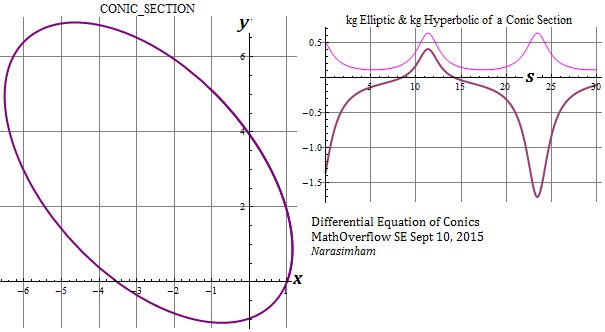
By choosing polar coordinates x,y symmetry is automatically built in it.

May I take opportunity to mention my earlier found differential equation of all conics in polar coordinates using isometric curvature invariants? The formulation is by differentiation of the latus-rectum of any conic

$$ \boxed{p\cdot k_{gE} = \sin^{3}\psi } \tag{1}$$

where $r$ is radius vector, $\psi$ is angle between $r$ and conic. $kg_E, kg_H$ elliptic and hyperbolic curvatures defined by Liouville's and my curvature formulas respectively as:

$$ kg_E, kg_H= \psi^{'} \pm\frac {\sin \psi}{r} \tag{2}$$

Primes are taken on arc of conic.

$$ \psi^{''}= 3 \cot \psi \cdot \psi^{'} \cdot kgE - \frac {\cos \psi}{r}\cdot kgH. \tag{3} $$

A conic is traced on the basis of the above, and both curvatures, elliptic and hyperbolic, are plotted.

EDIT 1:

Just after the above posting here it came to light that only elongated conics can have hyperbolic points of inflection. The limiting condition is that the eccentricity of a ellipse conic $ \epsilon \ge \frac12 $ to have points of local hyperbolic straightness; hyperbolas and parabolas would naturally qualify for this ( $ \epsilon \gt \frac12 $ ) ... am sharing this with you hoping it stays well within the scope of DEs of conics.

EDIT2:

Another most useful form of conics ode is

$$ \boxed{ \tan \psi \cdot \psi^{''} = (\frac {\sin \psi}{r} )^2 + 2 \psi^{'2} = 3 \psi^{'} \cdot kgE - \frac {\sin \psi}{r}\cdot kgH} \tag{4} $$

EDIT 3:

My earlier work regarding intrinsic differential equation of conics:

Mathforum__sci.math_Referece

EDIT4:

The ellipse below is computed and depicted from conics differential equation (1) I had derived earlier. Note that the its focus position is arbitrary not at origin , it is not a Newtonian ellipse rotated about focus.

EDIT 5

Conic sections arise by sectioning a right circular cone ($ \gamma\, = $semi vertical angle) with planes of variable inclination $\alpha.$ Differential equations of conics in their original/formative natural setting are shown below with direct comprehensive relevance in the 3-space.

Consider differential triangle $ \Delta\,MVR $

$$ \tan \gamma= \frac{MR}{VR}=\frac{y- r_{min}}{z-z_{min}} = \frac{r \sin \theta- r_{min}}{z-z_{min}} = \frac{dr}{dz}\tag{5/1}$$

$ {\psi,\gamma}$ are angles meridian makes to 3D conic arc and the symmetry axis respectively. Now differentiate using cylindrical coordinates priming differentials on arc length using differential relations:

Note: The same symbol for $\psi$ is used for denoting angle between radius-arc in the previous case when it is in the flat plane and between meridian-arc in 3D. This is strictly speaking incorrect. At a later date it would be however attempted to be reconciled with a new notation. It is temporarily retained because $(r-\psi)$ relations are invariant in $(kgE,kgH)$ above definitions valid in 2D and 3D as for example $ r \sin \psi = c $ is a geodesic invariant in either case.

$$r'= \sin \phi \cos \psi\,; \theta^{'}= \frac{\sin \psi}{r} \,;z'= \cos \phi \cos \psi; \tag{5/2}$$

Simplify and what we obtain appears to be a pure geometric relation between two variables $ (\psi,\theta):$

$$\tan \alpha \cos \gamma = \tan \psi \cos \theta+ \sin \gamma \sin \theta \tag{5/3} $$

This however is a first order ordinary differential equation if we bear in mind differential relations (1) which formed relations (5/2), but it is not merely a trigonometrical relationship.

The initial condition $ \theta =0 $ at point $P$

$$ \tan \psi= \tan \alpha \cos \gamma \tag{5/4}$$

It is known

$$\epsilon= \dfrac{\cos \alpha}{\cos \gamma}\tag{5/5}$$

(5/4) and (5/5) supply boundary conditions $\psi_i$ suitable to generate any choice of conic with eccentricity $\epsilon$.

$$ \tan \psi_i= \sin \alpha/\epsilon \tag{5/6} $$

Writing the conics differential equation instead of arc, in terms of $\theta$ and representing differentials with dots (instead of dashes) we have

$$ \boxed{\dot{\psi} = \cos^2 \psi ( \tan \psi . \tan \theta -\sin \gamma )} \tag{5/7}$$

The relations are integrated together and displayed below:

Answer 4

I think your original 5th order differential equation and the 5th order differential equation in the references cited by Dave Renfro can be partially understood without calculation.

What matters is that the family of conics is a 5-parameter family of 1-dimensional submanifolds in the plane $P$. The dimension of the total space of the family is 6, which is the same as the dimension of the space $P^{(4)}$ of 4-jets of curves in the plane. Stated correctly, it turns out that forming 4-jets of conics defines a bijection between these 6-dimensional manifolds. Forming their 5-jets yields a section $P^{(4)}\rightarrow P^{(5)}$. Its image $E$ is the ``geometric" expression of the differential equation.

Using this line of reasoning one can show that

1. A plane curve is a conic if and only if it makes order 5 contact with all of its (4th order) osculating conics.
2. The differential equation is equivalent to the condition that the curve make order 5 contact with its osculating conics at each point.

The different versions of the differential equation are probably just different functions cutting out the same zero set $E$ in $P^{(5)}$.

Answer 5

Let me try to give a semi-conceptual answer, which is still not free of some computations. It is inspired by beautiful book "Projective Differential Geometry Old and New: From the Schwarzian Derivative to the Cohomology of Diffeomorphism Groups" by Ovsienko and Tabachnikov.

The main idea is that there exists a correspondence between curves in $\mathbb{RP}^n$ and differential operators $$ A=\frac{d^{n+1}}{dx^{n+1}}+a_{n-1}(x)\frac{d^{n}}{dx^{n}}+\ldots+a_1(x)\frac{d}{dx}+a_0. $$ Projectively equivalent curves correspond to the same operator. Various interesting invariants, like so-called projective curvature, could be obtained from the coefficients of $A$. In our case we are given a curve $\gamma$ in $\mathbb{RP}^2$ with parametric presentation $$ [y(x):x:1], $$ which corresponds to an operator $$ A_{\gamma}=\frac{d^{3}}{dx^{3}}+a_{1}(x)\frac{d}{dx}+a_0. $$ Tensor density $A_0=(a_0(x)-\frac{1}{2}a_1'(x))(dx)^3$ is invariant under parametrization of the curve and is known to vanish if and only if the curve is a conic.

Here is a construction of operator $A_{\gamma}.$ Let's pick a lift $\Gamma(x)$ of our curve to $\mathbb{R}^3$ such that $$ \det (\Gamma, \Gamma', \Gamma'')=1. $$ Then for some functions $a_0, a_1$ we have a linear dependence $\Gamma'''+a_1 \Gamma' +a_0\Gamma.$ These functions are the coefficients of $A_{\gamma}.$

There exists a function $u(x)$ such that the lift of our curve equals to $(u y,u x,u ).$ An easy computation with determinant shows that $u=(y'')^{-\frac{1}{3}}.$

We know that functions $u$ and $xu$ are annihilated by operator $A_\gamma.$ This implies that $$3u''+a_1u=0.$$ Since $A_0 u=0,$ $$ u'''+a_1u'+a_0 u=0. $$ From these equations it is easy to see that $$a_0-\frac{1}{2}a_1'=0$$ if and only if $$(u^2)'''=((y'')^{-\frac{2}{3}})''' =0.$$