1
$\begingroup$

Let $f,g$ be two polynomials in $\mathbb{Z}[x,y]$, given by $$ f(x,y)=x^4-3xy+y^2,$$

$$ g(x,y)=x^5-4xy+3xy^2.$$

Let $I=(f,g)$ be the ideal in $\mathbb{Z}[x,y]$ generated by $f$ and $g$.

Is $x,x^2,x^3,x^4,x^5,x^6,x^7,x^8, x^9, x^{10}, x^{11}$ lies in $I$ respectively?

Improve this question
$\endgroup$
4
  • 1
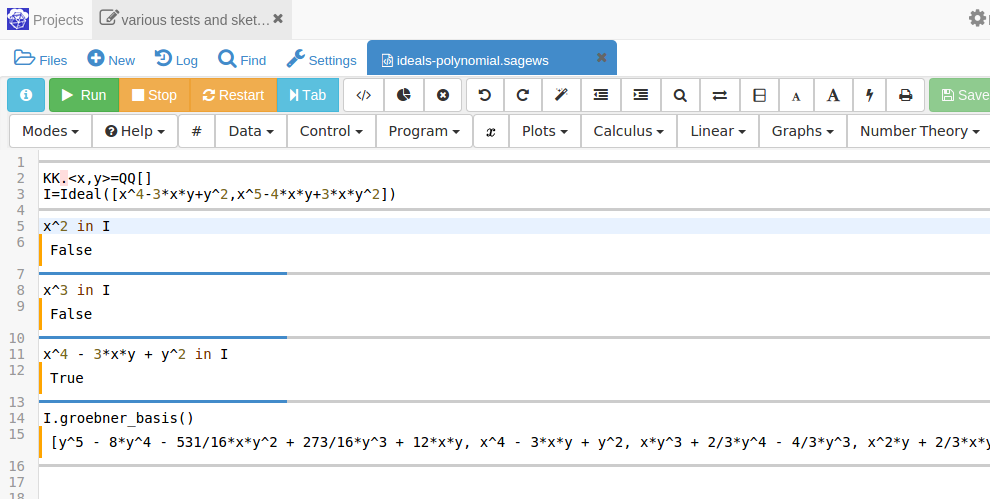
    $\begingroup$ I believe you can solve this in sage, possibly online with a browser. Session: KK.<x,y>=QQ[];I=Ideal([x^4-3*x*y+y^2,x^5-4*x*y+3*x*y^2]);x^2 in I $\endgroup$
    – joro
    Commented Apr 23, 2015 at 9:48
  • $\begingroup$ thanks so much! could you give me the programme website? $\endgroup$
    – Shiquan Ren
    Commented Apr 23, 2015 at 11:38
  • 1
    $\begingroup$ Sure, just to make try to export the web example. Sage is free software: sagemath.org. If your platform isn't supported, you can run it locally in virtual machine. $\endgroup$
    – joro
    Commented Apr 23, 2015 at 12:15
  • 1
    $\begingroup$ For browser, create account on: cloud.sagemath.com. Create sage worksheet and paste my example, then choose |RUN|. Better replace ";" by newline, newlines are non-trivial in comments. $\endgroup$
    – joro
    Commented Apr 23, 2015 at 12:20

1 Answer 1

Reset to default
3
$\begingroup$

Extending on @joro's answer: open a public sagemathcloud worksheet

KK.<x,y>=QQ[]
I=Ideal([x^4-3*x*y+y^2,x^5-4*x*y+3*x*y^2])
x^2 in I

sagemathcloud polynomial ideal

Improve this answer
$\endgroup$
1
  • $\begingroup$ How did you make it public? $\endgroup$
    – joro
    Commented Apr 25, 2015 at 7:05

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .