1
$\begingroup$

I googled for papers on applying algebraic topology to 3d/4d printing. It just seems to me that there has to be a connection. Any help, kind audience?

edit: 4d printing means 1-parameter families of 3d printed objects. Movies with 3d printed objects, one object per frame.

Improve this question
$\endgroup$
9
  • 3
    $\begingroup$ The title refers to "printing" (what's 4d printing?), but the body of the question does not. What are you looking for? I don't think the question can be given a useful answer without more details. $\endgroup$
    – Henry Cohn
    Commented Jan 21, 2015 at 17:29
  • 2
    $\begingroup$ Why do you insist on algebraic topology? How exactly did you come to this idea? $\endgroup$
    – Alex Degtyarev
    Commented Jan 21, 2015 at 17:42
  • 1
    $\begingroup$ @HenryCohn Presumably, 4D printing is 3D printing with programable shape transformation (i.e., the time axis gives the 4th dimension) like this: ted.com/talks/… $\endgroup$
    – Yuichiro Fujiwara
    Commented Jan 21, 2015 at 18:18
  • 2
    $\begingroup$ Thanks for the link! My first thought was that it was the analogue of 3d printing for people who live in $\mathbb{R}^4$. $\endgroup$
    – Henry Cohn
    Commented Jan 21, 2015 at 18:24
  • 3
    $\begingroup$ This feature article from AMS explores some mathematics used in 3D printing, but the involved mathematics is related to computational geometry, not algebraic topology. $\endgroup$
    – Tadashi
    Commented Jan 21, 2015 at 18:25

2 Answers 2

Reset to default
7
$\begingroup$

There are certainly many "digital artists" who are inspired by topology in their 3D-print designs. E.g., Torolf Sauermann:
               
                (Image from this web site.)

Improve this answer
$\endgroup$
2
6
$\begingroup$

I think one excellent 4d printing project that (to my knowledge) nobody has done yet would be to print the Optiverse. This is John Sullivan's minimal elastic bending energy version of the $\mathbb RP^2$-midpoint Shapiro-Morin sphere eversion.

http://www.math.uiuc.edu/~jms/Papers/isama/eversions.pdf

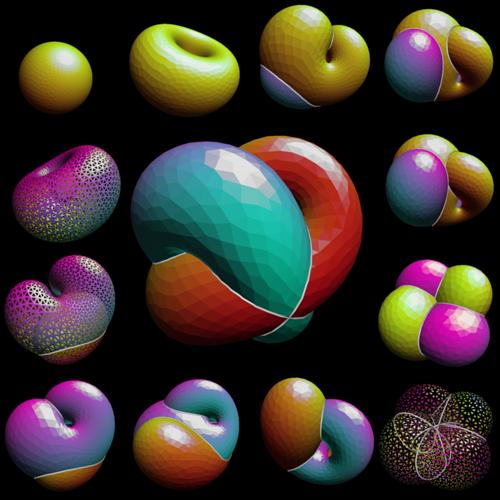
enter image description here

This would take quite a bit of work as you would have to make some fairly intelligent choices of how to make cuts in the surface in order to see what's going on, yet not lose track that this is a sphere.

But who knows, maybe Sullivan has a version of this on his office desk? It wouldn't surprise me.

Improve this answer
$\endgroup$

Not the answer you're looking for? Browse other questions tagged .