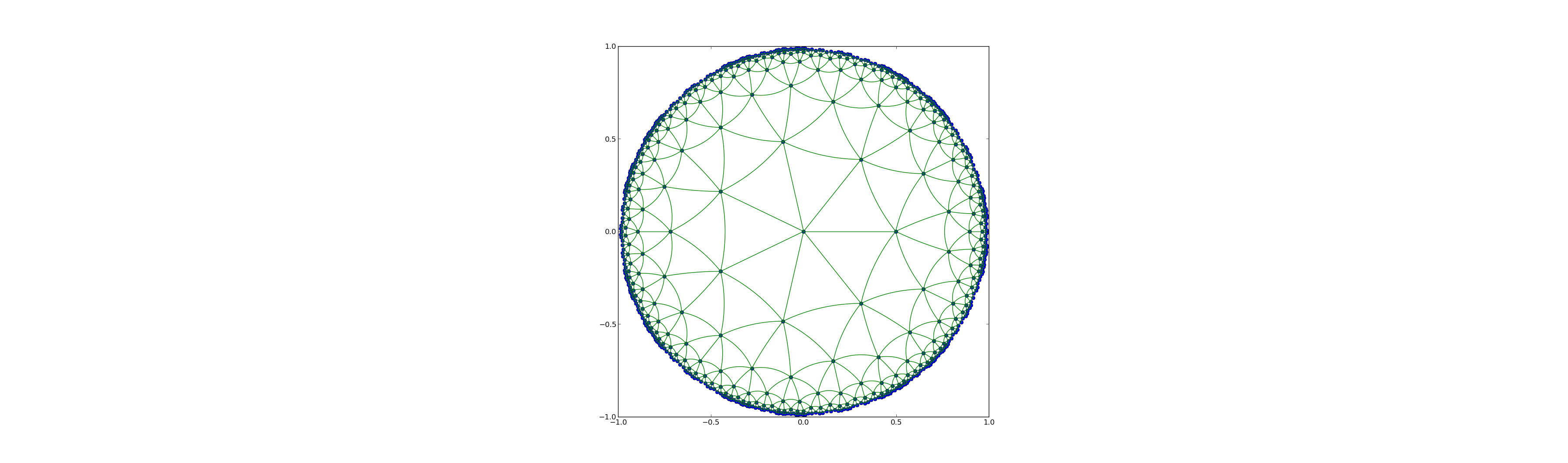
Let $T_d$ be the infinite valence $d$ triangulation of the hyperbolic plane, where each triangle is equilateral and $d \ge 7$. Question: Is there an isometric embedding from $T_d \to \mathbb{R}^3$? Here an isometric embedding means that each triangle is flat, with Gaussian curvature only at the vertices.
I've been told that Thurston and others have tackled this problem and failed, so apparently it is fairly hard. Actual question: Are there references discussing existing attempts?
The problem is likely very different for even vs. odd $d$, since for even $d$ we can almost fold along geodesics down to a single triangle. That is, given any $\epsilon > 0$, there is an almost isometric embedding of $T_{2d}$ into an $\epsilon$ neighborhood of a single triangle, where the isometry is off by a factor of $1 \pm \epsilon$.
For odd $d$, at least, my intuition is that it is completely unreasonable that such an embedding exists: the number of triangles in a ball grows exponentially with the radius due to the exponential growth of hyperbolic space, which results in an exponential number of arbitrarily closely layered sheets.
Here is the radius 5 portion of $T_7$ in the Poincare disk model, together with an embedding into $\mathbb{R}^3$: