In 2 hours after posting this, I realized that preserving Liouville measure solves the problem completely. Sorry for disturbing...
Construction of perpetuum mobile: Consider room with mirror walls formed by two arcs of two ellipses with common foci and two segments of on bisecting perpendicular for the focuses as on the picture:
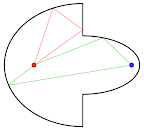
Place one-point-bodies with the same temperature in each focus --- they radiate and
All rays from blue focus come to the red one.
Big portion of rays from red focus goes back to it-self (that are all rays which reflect in bisector) while the rest goes to the blue focus.
Thus red focus getting hotter than blue one; i.e., we have a perpetual motion machine of the second kind...
Questions:
Why exactly it does not work? My guess is: if instead of one-point-bodies we have bodies with real size (no matter how small) it will no longer work, but I'm too lazy to do calculations, and I also it should be a good explanation (with no calculations).
For those of you who think it is not math, here is math formulation: Assume instead of one-point-bodies we have very small bodies of arbitrary shape. Then physics tells us it should not longer work. BUT I do not see mathematical proof of it...
Comment:
- I know this construction from Vladimir Troitsky. A similar (but not as elegant) construction appears in comment on the Brain Teaser in the September 1972 issue of Physics Education (page 414). (maybe earlier?) --- thanks to Scott Carnahan for the ref. On page 446, there is a "solution", it only says that "it would not work because of the finite sizes of [the bodies]". Next year (June 1973, p.292) a letter with a better explanation was pubilshed in the same journal, this "better explanation" roughly says that it does not work by a "well established law".
