Here is a Bratelli-Vershik graph: alt text http://www.freeimagehosting.net/newuploads/64jrg.jpg
This graph should (I do not master this topic) define a Vershik adic transformation $T$ on its path space.
What are the invariant measure(s) on the path space for which $T$ is ergodic ?
Is $T$ (isomorphic to) a known example of transformation in ergodic theory ?
EDIT 26/08/2012: I finally don't understand why $T$ is isomorphic to the dyadic odometer, as claimed in RW's answer below. Below are some observations about this graph.
To be sure of the definition of the graph, using Vershik terminology it is given by the Markov compactum $(M_n)$ where $M_n$ is the $n\times (n+1)$-matrix obtained by concatenating the $n \times n$-identity matrix with a column of ones: $$M_n=\begin{array}{cccccc} 1 & 0 & \cdots &\cdots & 0 & 1 \\\ 0 & \ddots & \ddots & & \vdots & \vdots \\\ \vdots & \ddots & \ddots & & \vdots & \vdots \\\ \vdots & & & & 0 & \vdots \\\ 0 & \cdots & & 0 & 1 & 1 \end{array}$$
In figure below this is an example of the action of $T$, the blue path becomes the red path:
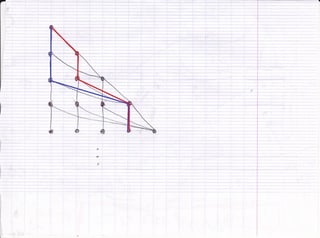
- According to the link between adic and cut-and-stack the transformation $T$ should correspond to a cutting and stacking construction looking like:
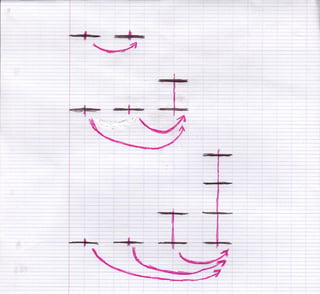
Is there something wrong in my understanding ? Could you elaborate a little the isomorphism between $T$ and the dyadic odometer ? Perhaps I could convince myself that the cutting and stacking construction asymptotically coincides with the odometer, but I don't see the isomorphism between the paths space of this adic graph and the paths space of the usual adic graph of the odometer.
EDIT (later): I have checked that the cut-and-stack procedure is indeed an approximation of the dyadic odometer. Hence RW is right but still I don't see the isomorphism between the paths space.
