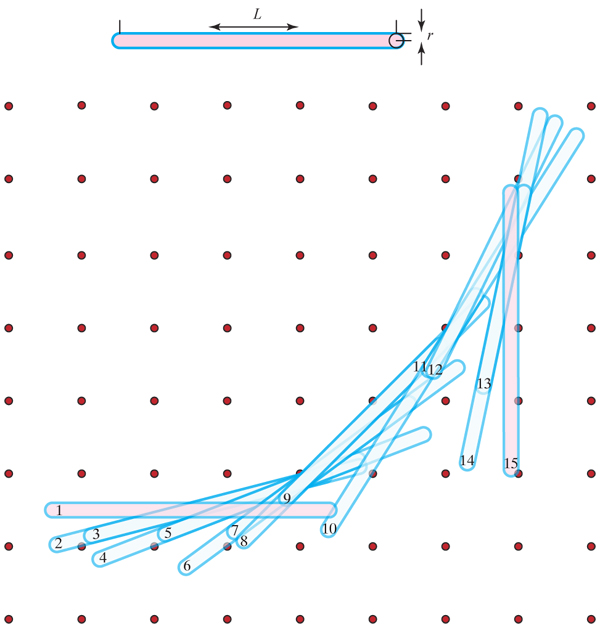
Imagine an object, which I'll call a ladder $\cal{L}$, a "racetrack" shape
composed of a rectangle of length $L$ capped at either end by
semicircles of radius $r$; so it is $L+2r$ tip-to-tip.
View every lattice point of $\mathbb{Z}^2$ as a point obstacle
(a pole). The ladder is forbidden to include a pole in its
interior, but $\cal{L}$ may touch poles on its boundary.
$\cal{L}$ is initially sitting in the plane in a horizontal orientation.
My question is:
Q. Under what conditions on $L \ge 0$ and $r \le \frac{1}{2}$can $\cal{L}$ be reoriented via continuous motions to a vertical orientation?
Throughout the motions, no pole may be interior to $\cal{L}$.
If $L=0$, $\cal{L}$ is a disk of radius $r$; if $r=0$, $\cal{L}$ is a segment of length $L$.
I believe that, for $r=0$, there is no upper bound on $L$: an arbitrarily
long segment can be reoriented.
(I made this an exercise (7.3) in a
textbook.)
And certainly when $r=\frac{1}{2}$, and, say $L=1$, reorientation
is not possible.
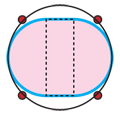
I can see that, for $r=\frac{1}{2}$,
any $L \le \sqrt{2}-1$ can be reoriented
(because then $\cal{L}$ fits inside a circle of radius $\sqrt{2}/2$).
Almost everything else is unclear to me.
An analogous question may be posed for an appropriately defined "ladder" amidst $\mathbb{Z}^d$ obstacles.
Any insights, even for specific $(L,r)$ values, or corrections to my beliefs above, are welcomed. Thanks!
