18
$\begingroup$

The question arose this morning during a seminar about HAs.

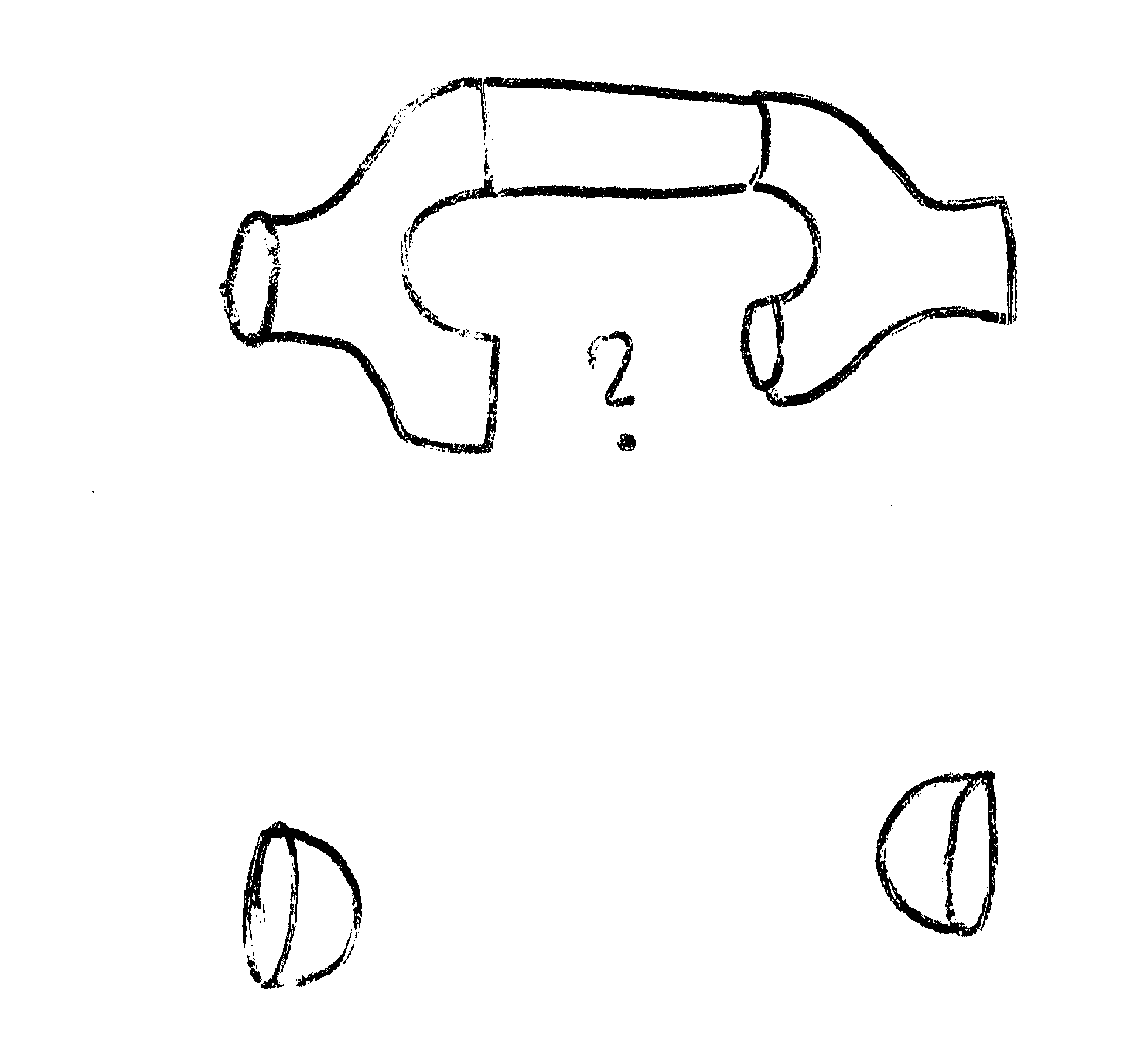
In a few words: can the equivalence $2-TQFT_k \leftrightarrow Frob_k$ be "modified" in a sensible way to give a similar one between the category $HA$ of Hopf algebras and a suitable "topological" category (I mean: a -even functor- category made 'with' topological objects, hopefully in a sufficiently small neighborhood of $2-TQFT$)? In particular i would like to find a visual analogue for the antipode map $s:H\to H$.

Bad thing is that it takes a while to discover there seem to be no way to define it as an arrow in $Cob(2)$: just try to draw in $Cob(2)$ the diagram

alt text

...any sensible choice for $s$ leaves in the manifold one hole more than the minimum. Spending a couple of words about the "sensible choice", it seems to me the only way not to increase the genus of the surface is to take as cobordism a-cap-and-a-cup, namely the [Cob(2)-analogue of the] composition $\eta\circ \epsilon\colon H\to k\to H$ in the former diagram... But I'm not able to characterize it as a Frobenius-Algebra map in any sensible way.

So, help me... (maybe the person I discussed with this morning is here? His website is this.)

Improve this question
$\endgroup$
8
  • $\begingroup$ I have seen what the relationship between the product and coproduct looks like in a bialgebra written out diagrammatically, and it just isn't very topological... $\endgroup$
    – Qiaochu Yuan
    Commented May 24, 2011 at 20:50
  • $\begingroup$ What do you mean by that? $\endgroup$
    – fosco
    Commented May 24, 2011 at 21:25
  • $\begingroup$ Super interesting question $\endgroup$
    – B. Bischof
    Commented May 25, 2011 at 14:13
  • $\begingroup$ Hello Do you check this article arxiv.org/pdf/hep-th/9412025.pdf It is a different approach, if you sen me your mail I can send you an exposition I prepared about this article. Best Carlos my mail is [email protected] $\endgroup$
    – Carlos Segovia
    Commented Jun 22, 2012 at 1:49
  • 1
    $\begingroup$ I just came upon this post, and was surprised not to find a discussion of the recent work of Douglas, Schommer-Pries, and Snyder. They explain that the fully dualizable objects in the 3-category whose objects are fusion categories, 1-morphisms are bimodule categories, 2-morphisms are bimodule functors, and 3-morphisms are natural transformations, are precisely fusion categories. Examples of fusion categories come from the representations categories of finite dimensional semi-simple Hopf algebras, and in this case the fusion categories you get are pivotal, which is a good categorification... $\endgroup$
    – David Jordan
    Commented Jun 22, 2012 at 2:12

3 Answers 3

Reset to default
8
$\begingroup$

Earlier than trying to handle the antipode map, you're dead in the water just trying to see the multiplication and comultiplication in a bialgebra as both corresponding to pairs of pants. Indeed, in a bialgebra you do not expect that

$$ X \otimes X \quad\overset{\Delta \otimes \operatorname{id}}\longrightarrow\quad X \otimes X \otimes X \quad\overset{\operatorname{id} \otimes m}\longrightarrow\quad X \otimes X $$

should have much to do with the map

$$ X \otimes X \quad \overset m \longrightarrow \quad X \quad \overset\Delta\longrightarrow \quad X \otimes X $$

whereas these are equal in a Frobenius algebra, because of the various ways to decompose the sphere with four punctures into two pairs of pants.

So I think the answer to your question is "no".

Improve this answer
$\endgroup$
6
  • $\begingroup$ Can't I flag both to be the answer? This is algebraically clear, and that provides a useful reference... :P $\endgroup$
    – fosco
    Commented May 25, 2011 at 11:51
  • $\begingroup$ But perhaps there are ways to add markings to the surfaces or otherwise limit the possible isotopies so that we don't expect those two maps to be equal? $\endgroup$
    – Sammy Black
    Commented May 25, 2011 at 19:24
  • $\begingroup$ @Sammy: Well, here's a stupid way that the answer is "yes". There is a "topological" category freely generated by two "Y" shapes (one-dimensional CW modules, or pairs of pants but with enough markings to make them behave essentially one-dimensionally), with markings or orientations or whatever you want so that one has two "inputs" and one "output", and the other is the reverse. Oh, also I want, if I'm thinking of these as surfaces, some half spheres that let me retract the Ys to tubes (or use a 1-dimensional model). Then if they have enough markings, you would not expect a (continued) $\endgroup$
    – Theo Johnson-Freyd
    Commented May 26, 2011 at 4:07
  • $\begingroup$ (continuation) condition like the one above to hold (which is good, since we're trying to present Hopf algebras). Ok, fine, so then you could just declare that through some magic, there is an isomorphism of these surfaces of whatever (e.g. find some distinguished cobordism-ish thing that realizes what I'm about to say, and mod out by cobordism-ish-es it generates) so declare that the "bialgebra compatibility condition" holds (this condition can be expressed as an equality of two different diagrams that can be made out of your Y-shaped generators). Now if you want, also give yourself (cont'ed) $\endgroup$
    – Theo Johnson-Freyd
    Commented May 26, 2011 at 4:10
  • $\begingroup$ (cont'ation) a marked interval which will play the role of the antipode, and just impose a relation like the one mentioned at in tetrapharmakon's answer below. There, you have a "topological" category for which functors out are Hopf algebras. But is it useful? NO! Or, anyway, not as such. It is useful to remember that many types of algebraic objects can be "presented" in terms of some rewrite rules on labeled graphs --- this is one of the big ideas in the theory of operads. But it does not help you study TQFTs or homotopy theory of manifolds. $\endgroup$
    – Theo Johnson-Freyd
    Commented May 26, 2011 at 4:12
4
$\begingroup$

I'd like to buy some hypotheses.

Hopf algebras are ubiquitous algebraic objects that can be the cohomology ring of an H-space, the Universal enveloping algebra of a Lie algebra, the group ring of a group, or a crucible for understanding a solution to the Yang-Baxter equation. Put on enough additional hypotheses and you can get several different theorems of the form you are asking for.

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ Here is a way to understand the antipode. The linear maps from the Hopf algebra to itself form an algebra under convolution. That is, $$ L*M(h)=m\circ(L\otimes M)\circ \Delta(h)$$ where $m$ is the multiplication and $\Delta$ is the comultiplication. The anditpode is the multiplicative inverse of the identity under this product. $\endgroup$
    – Charlie Frohman
    Commented May 25, 2011 at 0:32
  • 3
    $\begingroup$ Finally, this might be pertinent; [38] Non-involutory Hopf algebras and 3-manifold invariants. Duke Math. J. 84 (1996), 83-129, arXiv:q-alg/9712047 , MR 1394749 (97g:57021) $\endgroup$
    – Charlie Frohman
    Commented May 25, 2011 at 0:53
4
$\begingroup$

This is my "geometric freshman explanation":

the problem is to put something instead of the "?" doing the job of $s$ in

enter image description here

...but the composition $\eta\circ\epsilon$ is disconnected, and there is no way to obtain a disconnected manifold starting gluing something to that. :( such a pity.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Can the Dehn twist fill the role of the antipode here? $\endgroup$
    – Bob
    Commented Oct 3, 2018 at 18:25

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .