Given a closed convex set $K\subset \mathbb{R}^d$ and a point $x\in K$ the tangent cone to $K$ at $x$ is defined by \begin{equation} T_xK:=\overline{\{v\in \mathbb{R}^d: \exists \lambda \geq 0 \text{ and } \exists y \in K\text{ such that }v=\lambda (y-x)\}}. \end{equation} (here, the overline $\overline{}$ denotes the closure, this is one possible definition, many others can be found, for more general classes of sets, but we always restrict ourselves to $K$ convex).
Examples: if $x\in \text{Int}(K)$ then $T_xK=\mathbb{R}^d$, $T_a{\{a\}}=\{0\}$
Now take $K:=\bar{U}, U$ is an open, connected, convex subset of $\mathbb{R}^d.$ Let $x\in \bar{U}.$ I'd like to know and explore the symmetries of the tangent cone $T_x{\bar{U}}$ in this case.
In this regard, let's define: an axis of symmetry is a line $l$ or equivalently a vector $v$ in in the ambient Euclidean space $\mathbb{R}^d$ with respect to which the reflection $R_l$ leaves $T_x\bar{U}$ invariant, i.e. if $x\in K \iff R_l(x) \in K.$ cf: to my earlier question.
The following is clear so far:
When $x\in U$ is an interior point, the tangent cone $T_x{\bar{U}}$ is all of $\mathbb{R}^d,$ thus it got $d$ axes of symmetry (any orthonormal basis with $x$ as the 'origin' will work)
When the boundary $\partial{U}$ is smooth, $T_x\bar{U}$ is isometric to $\mathbb{H}^d,$ the closed upper half space. So it has $d-1$ axes of symmetry (any orthonormal basis of $\mathbb{R}^d \times \{0\}\subset $\mathbb{H}^d$ works), one axis missing from the last example, as along the inward normal direction, it's not symmetric anymore, since reflection w.r.t. that direction will map a point outside the domain.
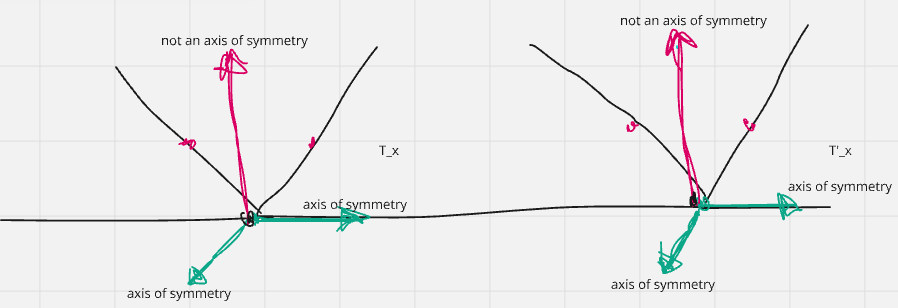
When the boundary $\partial{U}$ is not smooth, in some cases we can still have $d-1$ axes of symmetry, e.g. consider $U$ to be the first quadrant in $\mathbb{R}^2$ times $\mathbb{R}.$ See the drawing below:
In this case, $T_x\bar{U}$ still have $d-1=3-1$ axis of symmetry.
I'm interested in knowing the cases of non smooth open, connected, convex domains $U\subset \mathbb{R}^d$ we can have $d-1$ axes of symmetry, and when can we have less symmetry?
Thoughts: I tend to think to find the missing degree of symmetry, we need to first come up with a concept of "angle bisector" in higher dimensions, but I'm not sure: this may be the direction the symmetry will be missing. I also think it'll all come down to local symmetry of of $U$ at $x,$i.e. symmetry of $B(x;r)\cap U$ for some $r>0.$
P.S. References very highly appreciated!
P.S. 2: Please tell me if my question is unclear. I'm yet to come across a literature that specifies axis of symmetry and degree of symmetry, even though we intuitively know them. This is why I attempted to define it in here and in the linked post.