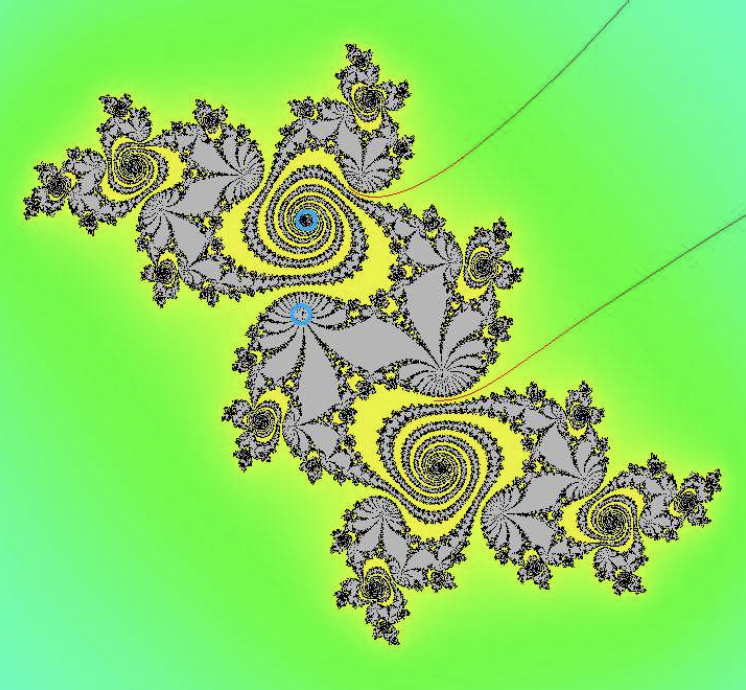
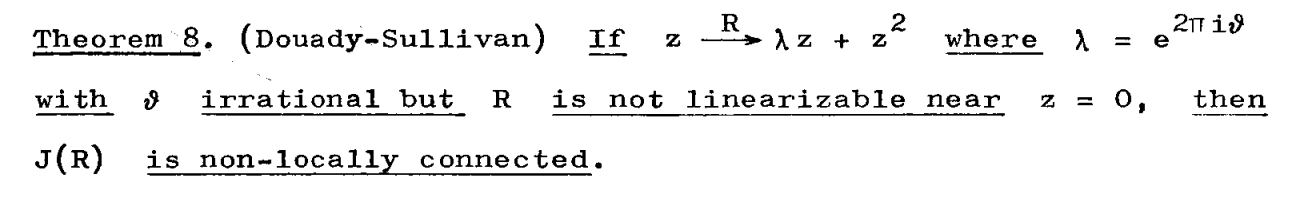What are some examples of complex polynomials whose Julia sets are connected, but not locally?
In the book Complex Dynamics by Carleson and Gamelin, I found: 
But what is a specific value of $\lambda$ that satisfies the hypotheses?
Ultimately I want to generate pictures of non-locally connected Julia sets, so I'm interested in any polynomials (in addition to $\lambda z+z^2$) with this property.