In the following proof (from The pontrjagin numbers of an orbit map and generalized G-signature theorem by Hsu-Tung Ku & Mei-Chin Ku https://link.springer.com/chapter/10.1007/BFb0085610), it is claimed that $\pi _{1}^{\ast }\left( z\right) $
is $H^{\ast }\left( B_{G};%
%TCIMACRO{\U{211a} }%
%BeginExpansion
\mathbb{Q}
%EndExpansion
\right) $-free. But I couldn't see why this was true.
$\begingroup$
$\endgroup$
9
-
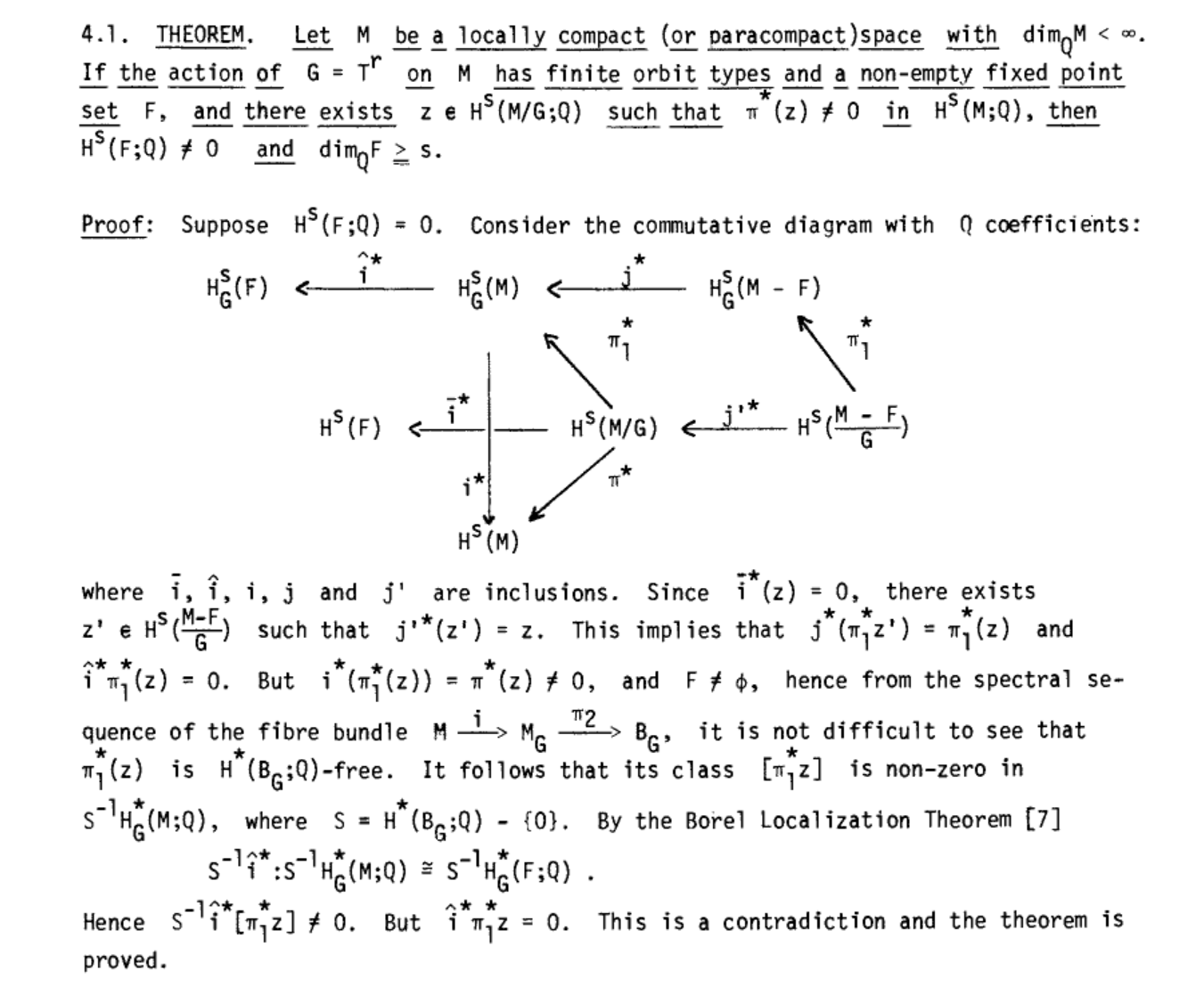
3$\begingroup$ I'm confused way earlier, doesn't the inclusion $j$ go $j: M-F\to M$, hence on cohomology, $j^*: H^s(M)\to H^s(M-F)$? $\endgroup$– Achim KrauseCommented May 17 at 8:22
-
$\begingroup$ @AchimKrause Yes you are right I didn't notice that. $\endgroup$– Mehmet OnatCommented May 17 at 8:28
-
1$\begingroup$ In the long exact sequence of a pair, shouldn't we identify the subspace to a point rather than remove it altogether? Otherwise, there isn't a map, or if there is, it goes the wrong way. $\endgroup$– Dave BensonCommented May 20 at 20:34
-
2$\begingroup$ When quoting someone else's work, please provide a proper citation, including names of authors and the title of the publication. $\endgroup$– John PalmieriCommented May 20 at 21:13
-
1$\begingroup$ Please put the citation information in the question itself, so that people don’t have to search the comments for it. The goal is to give appropriate credit to others for their work. $\endgroup$– John PalmieriCommented May 22 at 14:32
|
Show 4 more comments
1 Answer
$\begingroup$
$\endgroup$
3
I believe a key point is that the assumption that $F \neq \emptyset$ lets one know that the map $\pi_2: M_G \rightarrow B_G$ has a section, and thus the ring homomorphism $$ \pi_2^*: H^*(B_G) \rightarrow H^*(M_G)$$ is monic.
The way the proof is worded suggests that, in the $E_{\infty}$ page of the Serre spectral sequence computing $H^*(M_G)$, we can then deduce that the $H^*(B_G)$-module generated by $E_{\infty}^{0,*}$ will be a free $H^*(B_G)$-module. [Then the Borel localization theorem part of the argument would kick in.]
This freeness result would be clear if we could conclude that the spectral sequence collapses, but I am not sure I believe that just having that section lets us know this.
-
1$\begingroup$ Isn't the $H^*(B_G)$-module generated by $E_{\infty}^{0,*}$ already a free $H^*(B_G)$-module? $E_2^{*,0}=H^*(B_G)$ and the multiplication is given by $E_{2}^{p,0}\otimes E_{\infty }^{0,q}=E_{\infty }^{p,0}\otimes E_{\infty }^{0,q}=E_{\infty }^{p,q}\subset H^{p+q}\left( M_{G}\right) $? $\endgroup$ Commented May 22 at 9:11
-
1$\begingroup$ @MehmetOnat Why do you think that the map $E_{\infty}^{p,0} \otimes E_{\infty}^{0,q} \rightarrow E_{\infty}^{p,q}$ should be an isomorphism? $\endgroup$ Commented Jun 3 at 22:16
-
1