Bar construction in commutative algebras is calculated by pushout

Question

$\DeclareMathOperator\colim{colim}$ Also asked in MathStackexchange here

This is a statement in Lurie's Higher Algebra 5.2.2.4. 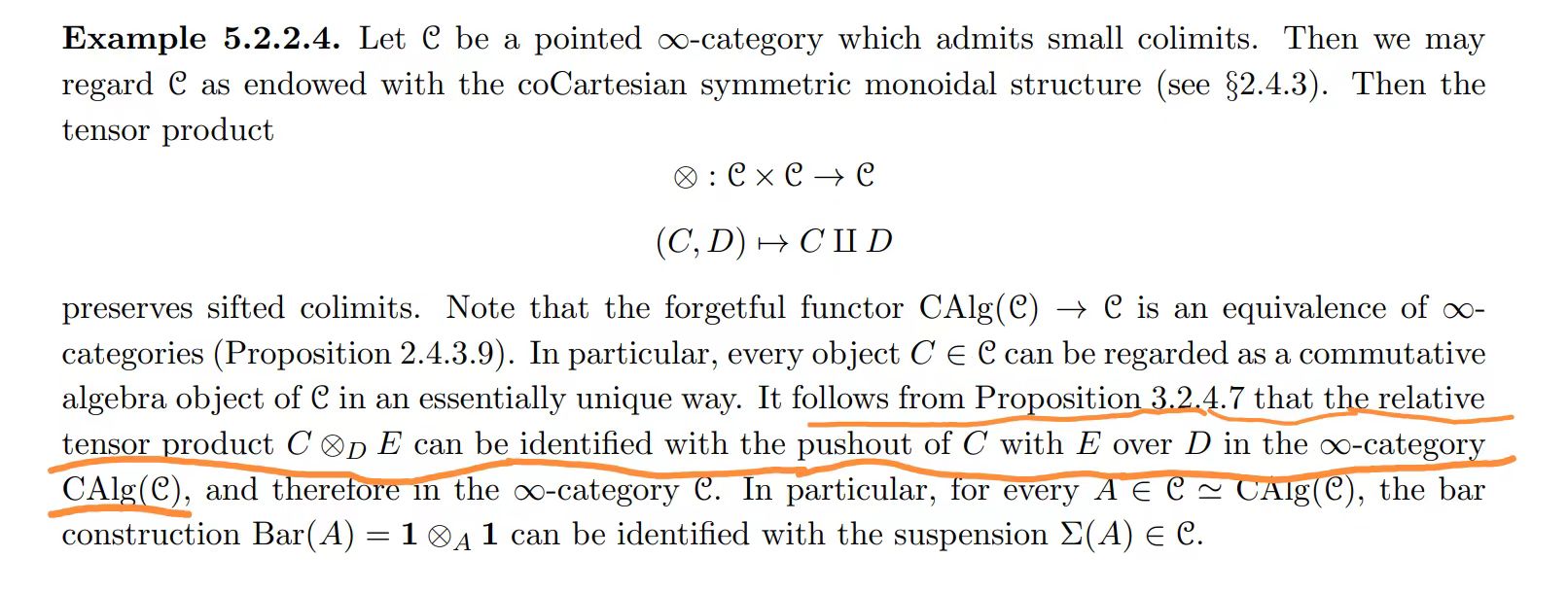Proposition 3.2.4.7 in HA said that the monoidal structure on $\text{CAlg}(\mathcal{C})$ is cocartesian. I don't know why this implies that the relative tensor product is identified with the pushout.

By [HA, 4.4.2.8], in a monoidal $\infty$-category $(\mathcal{D},1,\otimes)$, the relative tensor product is calculated by two-sided bar construction, i.e., $C\otimes_DE=\colim_{[n]\in\Delta^{\rm op}}C\otimes D^{\otimes n}\otimes E$
In our case $\mathcal{D}={\rm CAlg}(\mathcal{C})$, the tensor product is given by the coproduct, so we have $C\otimes_DE=\colim_{[n]\in\Delta^{\rm op}}C\coprod D^{\coprod n}\coprod E$

We need to show that $C\coprod_DE=\colim_{[n]\in\Delta^{\rm op}}C\coprod D^{\coprod n}\coprod E$ naturally. I have not found a good way to prove this.

Edit:
I have a similar question related to Gepner and Haugseng's "Enriched $\infty$-categories via non-symmetric $\infty$-operads"

They calculated a certain geometric realization, which turned out to be the homotopy pushout ${\rm pt}\coprod_X^h{\rm pt}$. I need more details about the "standard model-categorical approach" referenced above.

I think the two situations are essentially the same, but I don't know how to treat either case.

Answer 1

This is just to be explicit about the role of the bar construction in David's answer.

If $I$ is the category $a \leftarrow b \rightarrow c$ parametrizing pushout diagrams, then there is a functor $f: I \to \Delta^{op}$, given by the diagram $$ [0] \xleftarrow{d^1_0} [1] \xrightarrow{d^1_1} [0]. $$ Given any pushout diagram $g: I \to \mathcal C$, represented by $A \leftarrow B \rightarrow C$, we can take the left Kan extension of this diagram along $f$ to get a simplicial diagram $f_* g$ in $\mathcal C$. It has the same hocolim (hocolims are left Kan extensions along the map $I \to \ast$, and so this follows from the fact that left Kan extensions preserve composition). The pointwise formula for left Kan extension says that this simplicial diagram, in degree $n$, is $$ \mathop{\mathrm{hocolim}}_{f(i) \to [n]\text{ in }\Delta^{op}} g(i). $$ However, a careful examination of the index category decomposes as a disjoint union of categories: one for each surjection $[n] \to [1]$ in $\Delta$, and two for the maps $[n] \to [0]$ in $\Delta$ with preimages $a$ and $c$ in $I$. This ultimately leads to a decomposition of degree $n$ as $A \amalg B^{\amalg n} \amalg C$, and so this simplicial diagram also computes the homotopy pushout of $A \leftarrow B \to C$.

Answer 2

A way to see this which doesn't dive into the specifics of the simplicial diagram "$C\otimes D^{\otimes n}\otimes E$" is to apply 3.2.4.7 to the symmetric monoidal $\infty$-category $Mod_D(\mathcal C)$: the tensor product there is the relative tensor product $-\otimes_D -$, and using the equivalence $CAlg(Mod_D(\mathcal C))\simeq CAlg(\mathcal C)_{D/}$, you find that coproducts in $CAlg(Mod_D(\mathcal C))$ are exactly pushouts over $D$ in $CAlg(\mathcal C)$.

Answer 3

Welcome to MathOverflow! First, let me point out that what you're asking is already true at the 1-categorical level. The pushout in the category of commutative rings is computed by the tensor product. You can check this directly from the universal property. If $C$ and $E$ are commutative monoids in some symmetric monoidal category, then the pushout of $C\gets 0 \to E$ (which is of course the coproduct $C\coprod E$) is isomorphic $C\otimes E$ by the universal property of each construction. Similarly, the pushout of the span $C \stackrel{f}{\gets} D \stackrel{g}{\to} E$ is isomorphic to $C\otimes_D E$. To check that, write the latter as the coequalizer of $C\otimes D\otimes E \stackrel{\to}{\to} C\otimes E \to C\otimes_D E$. The two maps on the left are the action of $D$ on $C$ and $E$, e.g., $d\cdot e := g(d)*e \in E$. Coequalizing these two maps is the same as fitting universally in the pushout square associated to the span $C \stackrel{f}{\gets} D \stackrel{g}{\to} E$.

Since this proof only uses universal properties, it still works in the setting of Lurie's Higher Algebra. When you are looking at $C\otimes_DE=colim_{[n]\in\Delta^{\rm op}}C\otimes D^{\otimes n}\otimes E$, I don't think it's better to write it down in terms of the coproduct. Instead, just use the universal property on the former formulation, and use that on the right, $D$ is acting on both $C$ and $E$.

Moving on to the Gepner-Haugseng part of the question. Yes, the unreduced suspension $\Sigma X$ can be computed as a homotopy pushout, and the span is what you have indicated. Note that the pushout of that span is not the homotopy pushout. The "standard model-categorical approach" to compute the homotopy colimit of any diagram is as follows. First, view the diagram as an object in the projective model structure on the diagram category $M^D$. Second, cofibrantly replace the diagram in that model structure. This involves replacing the objects by cofibrant objects, the morphisms by cofibrations, plus more. Lastly, compute the colimit of that new diagram, and what you have is a homotopy colimit of the original diagram. This is all explained here, and in Emily Riehl's excellent book, among many other places. Example 6.4.6 in her book spells out the unreduced suspension as a homotopy pushout.