3
$\begingroup$

How do we compute the projective resolution of a representation of a quiver with relations.

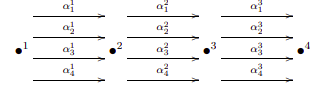
For example consider the Beilinson quiver $B_4$

enter image description here$.

with the relations ­$\{\alpha_j^k\alpha_i^{k-1}=\alpha_i^k\alpha_j^{k-1}:1\leq i,j\leq 4,1\leq k\leq 3\}$

Since the Beilinson quiver $B_4$ is derived equivalent to the $\mathfrak{D}^b(\mathbb{P}^3)$, it has finite projective dimension. How do we construct the projective resolution for a representation of this quiver?

I had first posted this question on stackexchage, but received no replies there. Thanks in advance!

Improve this question
$\endgroup$
5
  • $\begingroup$ In general, if there are no directed cycles, then the global dimension is finite. It is bounded by the length of the longest directed path. $\endgroup$
    – Dave Benson
    Commented Mar 18 at 9:22
  • $\begingroup$ Yes, I want to understand how exactly to construct the resolution. $\endgroup$
    – user52991
    Commented Mar 18 at 11:45
  • $\begingroup$ This boils down to (repeatedly) finding a (minimal) projective cover, which just means finding a minimal generating set. $\endgroup$
    – Hugh Thomas
    Commented Mar 18 at 17:41
  • 1
    $\begingroup$ If you have a particular representation in mind then you can calculate the projective resolution using the QPA package in GAP. They have command for calculating projective resolution. You can find more information about it here: gap-system.org/Packages/qpa.html $\endgroup$
    – It'sMe
    Commented Mar 18 at 18:51
  • 1
    $\begingroup$ Isn’t this Butler and King? sciencedirect.com/science/article/pii/S0021869398975998 $\endgroup$
    – Aaron Bergman
    Commented Mar 19 at 0:39

0

Reset to default

You must log in to answer this question.

Browse other questions tagged .