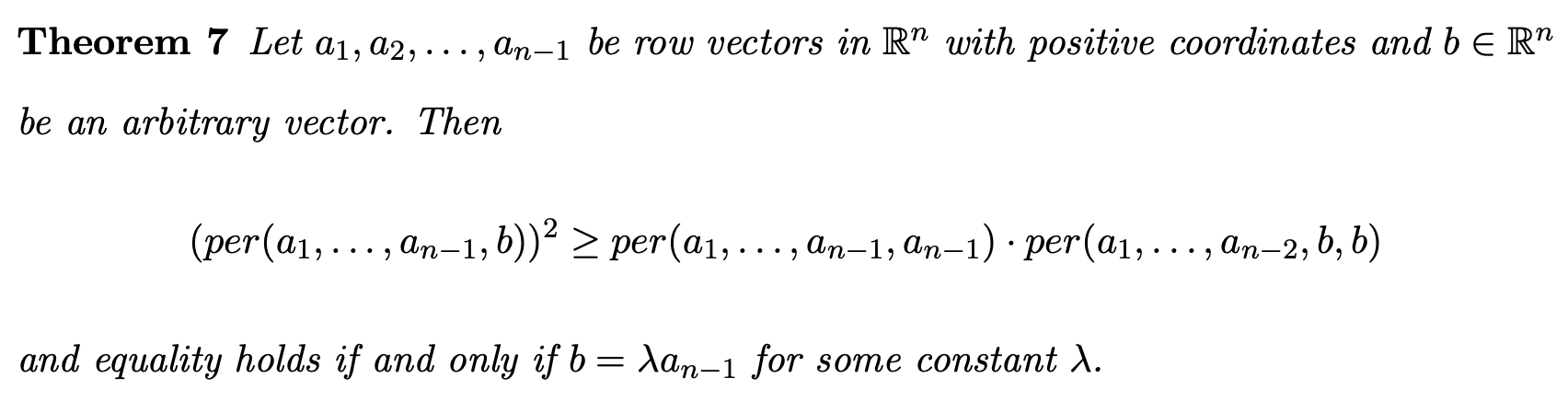1
$\begingroup$

The Van der Waerden conjecture is a lower estimate of the permanent of a doubly stochastic matrix. In this article in Wikipedia it is stated that Egorychev's proof uses the Alexandrov-Fenchel inequality for mixed volumes of convex bodies. Here is a link to the proof (which is very short). Egorychev himself mentioned the latter inequality but in a less direct way I think.

Where exactly the Alexandrov-Fenchel inequality is used in the proof?

I think what is actually used is Alexandrov's inequality for mixed discriminants of symmetric matrices which Alexandrov invented for one of his proofs of the Alexandrov-Fenchel inequality. But the former inequality is easier than and different from the latter.

Improve this question
$\endgroup$
3
  • 2
    $\begingroup$ the link to the proof appears to be incorrect. $\endgroup$
    – Mark Schultz-Wu
    Commented Mar 3 at 6:15
  • 1
    $\begingroup$ I think you yourself answered your question. $\endgroup$
    – Iosif Pinelis
    Commented Mar 3 at 13:32
  • $\begingroup$ @Mark: Correcred. $\endgroup$
    – asv
    Commented Mar 4 at 5:47

1 Answer 1

Reset to default
3
$\begingroup$

Egorychev’s proof of van der Waerden’s conjecture is dicussed by Martinez in Notes on the proof of the van der Waerden permanent conjecture (page 23). The Aleksandrov (1938) inequality needed is:

Historical note: This MO post argues that Van de Waerden did not actually formulate the conjecture that has his name.

Improve this answer
$\endgroup$
1
  • 1
    $\begingroup$ I don't see here an answer to the question "Where exactly the Alexandrov-Fenchel inequality is used in [Egorychev's]proof?" The answer is apparently "nowhere". $\endgroup$
    – Iosif Pinelis
    Commented Mar 3 at 13:30

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .