Suppose that topological group $G$ acting on topological space $X$. If the set $\left\{ \left[ G_{x}\right] :x\in X\right\} $ is finite, where $\left[ G_{x}\right] $ denotes the conjugacy class of the isotropy subgroup $G_{x}$ in $G$, then the group $G$ is said to act on a space $X$ with finitely many orbit types (FMOT). If the set $\left\{ \left[ \left( G_{x}\right) _{0}% \right] :x\in X\right\} $ is finite, where $\left( G_{x}\right) _{0}$ denotes the identity component of the isotropy subgroup $G_{x}$ in $G$, then the group $G$ is said to act on a space $X$ with finitely many connective orbit types (FMCOT).
I asked before that Fixed point set for a subcircle of torus actions if $T$ is a torus and $X$ is a $T$-space with finitely many connective orbit type, then there is a subcircle $L=\mathbb{S}% ^{1}\subset T$ such that $X^{L}=X^{T}$ (fixed point sets of the action). I couldn't get a complete answer to this question.
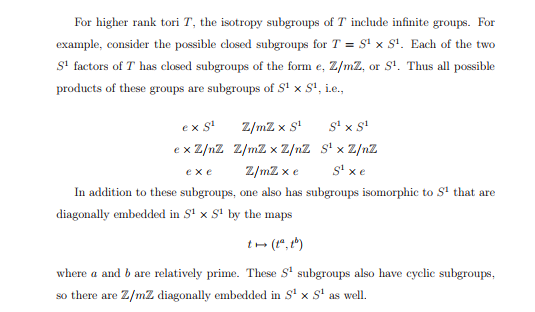
While I was thinking about this question, I came across something like this: Closed subgroups of torus $T=\mathbb{S}^{1}\times \mathbb{S}^{1}\times \cdots \times \mathbb{S}^{1}$ has type $\mathbb{S}^{1}\times \mathbb{S}% ^{1}\times \cdots \times \mathbb{S}^{1}\times %TCIMACRO{\U{2124} }% %BeginExpansion \mathbb{Z} %EndExpansion _{n_{1}}\times %TCIMACRO{\U{2124} }% %BeginExpansion \mathbb{Z} %EndExpansion _{n_{2}}\times \cdots \times %TCIMACRO{\U{2124} }% %BeginExpansion \mathbb{Z} %EndExpansion _{n_{k}}$. So connected closed subgroups of $T$ has type $\mathbb{S}% ^{1}\times \mathbb{S}^{1}\times \cdots \times \mathbb{S}^{1}\times \left\{ 1\right\} \times \left\{ 1\right\} \times \cdots \times \left\{ 1\right\} $. Isn't there already finite number of connective orbit types for torus actions on any topological space? Where am i doing wrong?
Add: I find this thesis ''Computation of Fixed Point Data from Equivariant Cohomology by Courtney K. Taylor''