When studying large systems of interacting particles, most of the work rely on mean field approximation by assuming that the number of particle goes to infinity. This allows to study the collective dynamics instead of following the particles. However, it seems there are very few references on the critical size(s) at which we observe the emergence of collective dynamics (or discussion on rate of convergence with respect to the number of particles)? Could anyone suggest some fundamental references (math, physics or experiments) that could shed some light on when the mean field approximation is appropriate or not? Thanks in advance.
$\begingroup$
$\endgroup$
2
-
$\begingroup$ There seems to be an implicit premise in this post that the mean field approximation becomes accurate for large systems. That is usually not the case. Since this approximation truncates correlations, its appropriateness critically depends on the type and strength of interactions rather than on system size, and has to be assessed case by case. $\endgroup$– Michael EngelhardtCommented May 1, 2023 at 4:48
-
$\begingroup$ I also find the characterization of the mean field approximation as describing collective dynamics questionable. Isn't the whole point of the approximation that one continues to treat the individual particle as an appropriate degree of freedom rather than passing to collective degrees of freedom? $\endgroup$– Michael EngelhardtCommented May 1, 2023 at 4:50
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
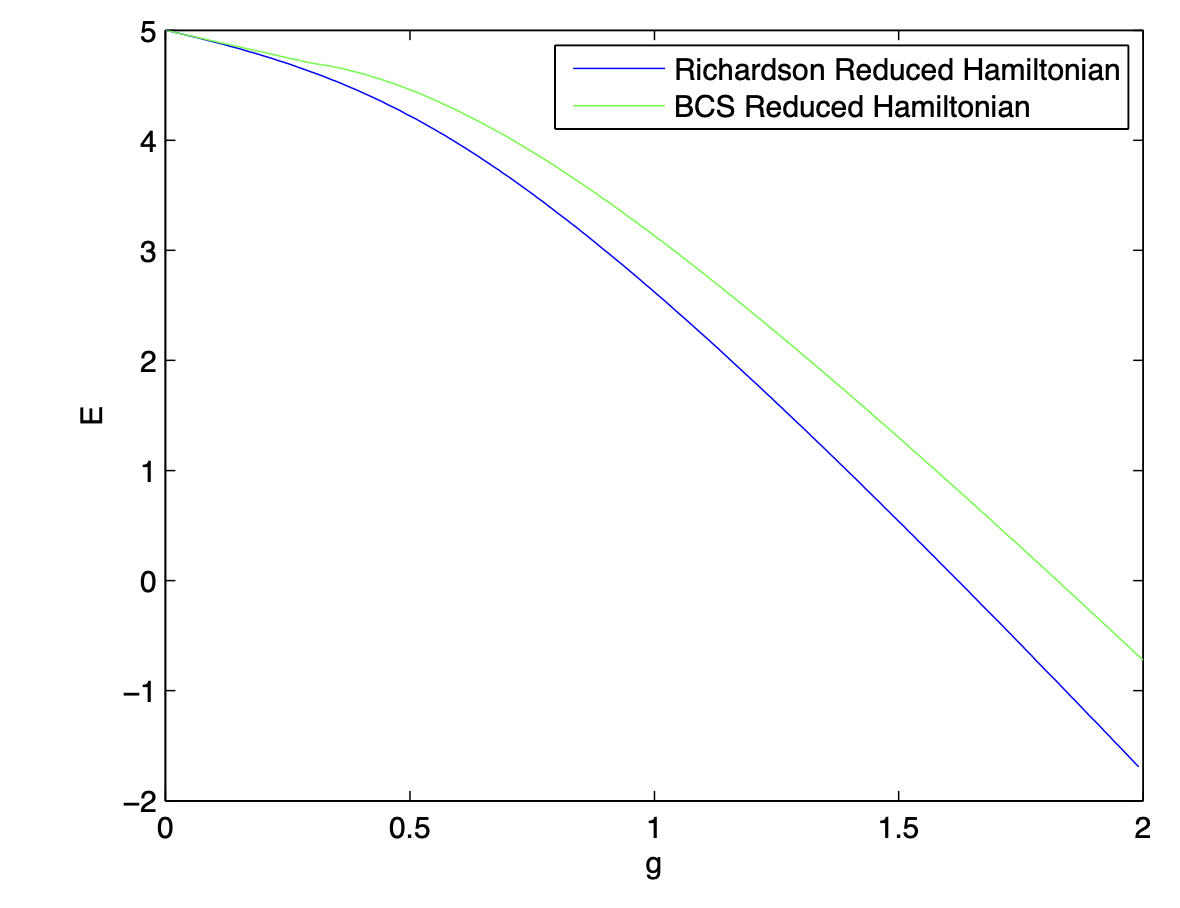
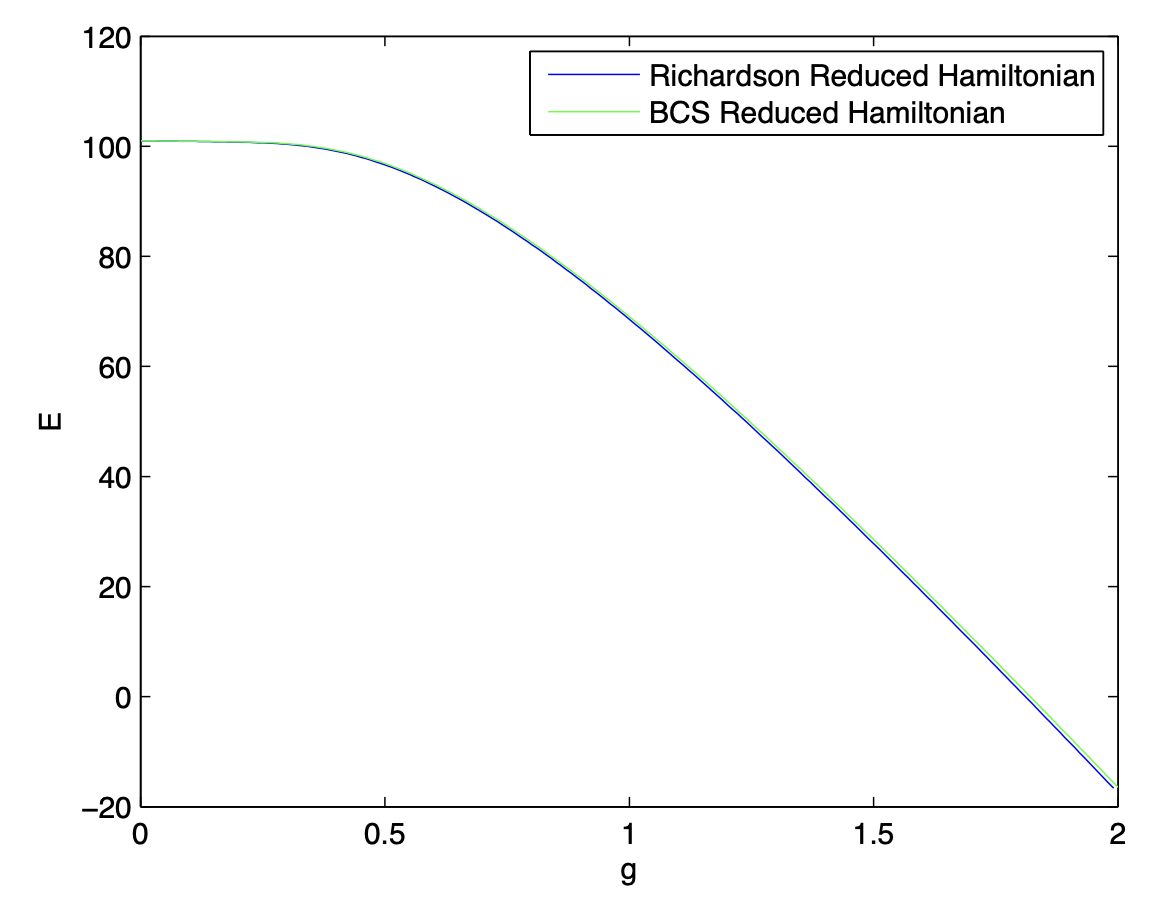
As pointed out in the comments, the mean-field approximation is not a $1/N$ expansion in the number of particles. Let me interpret the question a bit more generally, as asking for the accuracy of the mean approximation. One context in which this is studied in detail is superconductivity. The BCS (Bardeen-Cooper-Schrieffer) theory (1957) is a mean-field approximation, which can be compared with an exact solution of the ground state energy due to Richardson (1964).
The mean-field theory of superconductivity does not conserve particle number, so we do expect it to be less accurate for small $N$. Moreover it amounts to a linearization of quantum fluctuations in the energy, so we also expect it to be less accurate for larger interaction constant $g$. This is indeed what happens, see the plots (left for $N=8$, right for $N=200$).
answered May 1, 2023 at 6:16