I am currently working through the following paper:
Lapointe L., Lascoux A., Morse J.
Determinantal Expression and Recursion for Jack Polynomials
Electron. J. Combin. 7 (2000), Notes 1. DOI: 10.37236/1539
and I am having trouble reproducing something from that paper. This is of interest since it shows how one can express the Jack polynomial $J_{\kappa}^{(\alpha)}$ corresponding to an integer partition $\kappa$ as the determinant of an upper Hessenberg matrix (whose determinant is easy to evaluate), with the monomial symmetric polynomials as the basis.
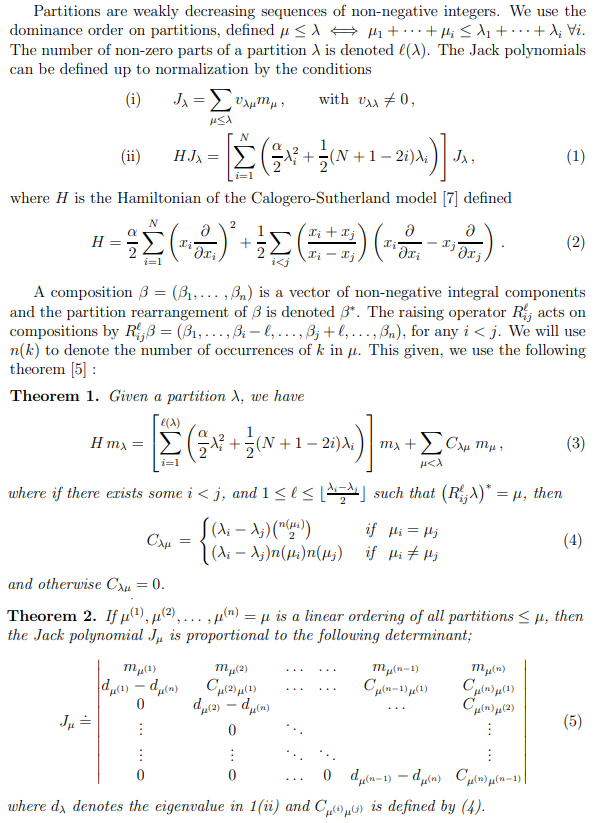
Here is the main result I am concerned with:
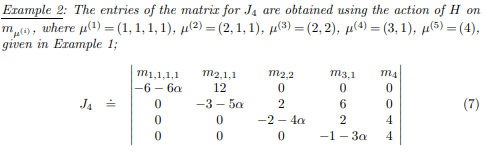
The paper proceeds with an example showing the Jack polynomial corresponding to the integer partition $(4)$:
My problem with the example above is in the entry $C_{\lambda\mu}$, where $\lambda$ and $\mu$ are respectively the integer partitions $(2\:2)$ and $(2\:1\:1)$. This corresponds to the $(3,3)$ entry of the determinant in the example. The paper shows that the number is $2$, but I am instead getting $4$ if I follow formula 4 in Theorem 1 that computes this number through the raising operator of a partition.
I find that $C_{\lambda\mu}=(\lambda_i-\lambda_j)n(\mu_i) n(\mu_j)=(2-0)\times 2\times 1=4$, since applying the raising operator $(R_{1,3}^{1}(2\:2\:0))^{\ast}$ (where we temporarily pad the integer partition with zeroes) does give $(2\:1\:1)$.
Did I misunderstand their Theorem 1? If, on the other hand, the formula for $C_{\lambda\mu}$ is in error, what can be done to fix it?
(Of note is that the example following Theorem 1 has a typo, which leads me to suspect that something has gotten omitted, but I have not been successful in finding an erratum for the paper, if there is one.)