Concurrencies determined by intersections of angle trisectors (and isogonal lines) in a triangle

Question

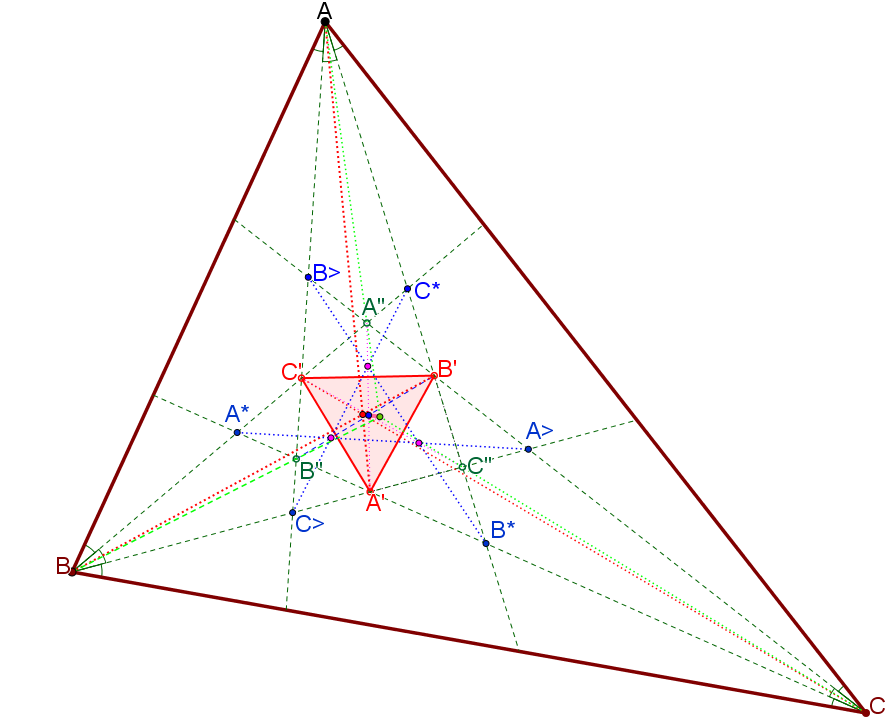
The famous Morley’s theorem, states that in a triangle the interior angle trisectors, proximal to sides respectively, meet at the vertices of an equilateral. However the six trisectors meet at 12 points. Denote the intersection of two trisectors with the letter of the vertex opposite to the common side of the angles to which these trisectors belong with a superscript. The intersections of the proximal and distal trisectors to sides are denoted by $A’$, $B’$, $C’$ (red) and $A”$, $B”$, $C”$ (green) respectively. Thus $\triangle A’B’C’$ is the Morley triangle. The 6 intersections (blue) each of a proximal and a distal trisector to a side are denoted by $A^>$, $B^>$, $C^>$ and $A^*$, $B^*$, $C^*$. Even though among $\triangle A’B’C’$, $\triangle A”B”C”$, $\triangle A^>B^>C^>$ and $\triangle A^*B^*C^*$ only the Morley triangle is equilateral, there are surprisingly many concurrencies between the lines joining corresponding intersections.

1. The lines connecting the corresponding intersections of proximal and distal trisectors meet at the same point (red), called 1st Morley center.
2. The lines connecting the corresponding vertices of the reference triangle $\triangle ABC$ and the Morley triangle $\triangle A'B'C'$ meet also at the same point (green) called 2nd Morley center.
3. Additionally the lines joining the corresponding vertices of $\triangle A’B’C’$ and $\triangle A”B”C”$ meet at the same point (blue) which is collinear with the 1st and 2nd Morley centers.
4. Moreover the lines connecting two pairs of corresponding vertices of $\triangle A^>B^>C^>$ and $\triangle A^*B^*C^*$ are concurrent with the line connecting the third pair of corresponding vertices of $\triangle A’B’C’$ and $\triangle A”B”C”$.

Questions

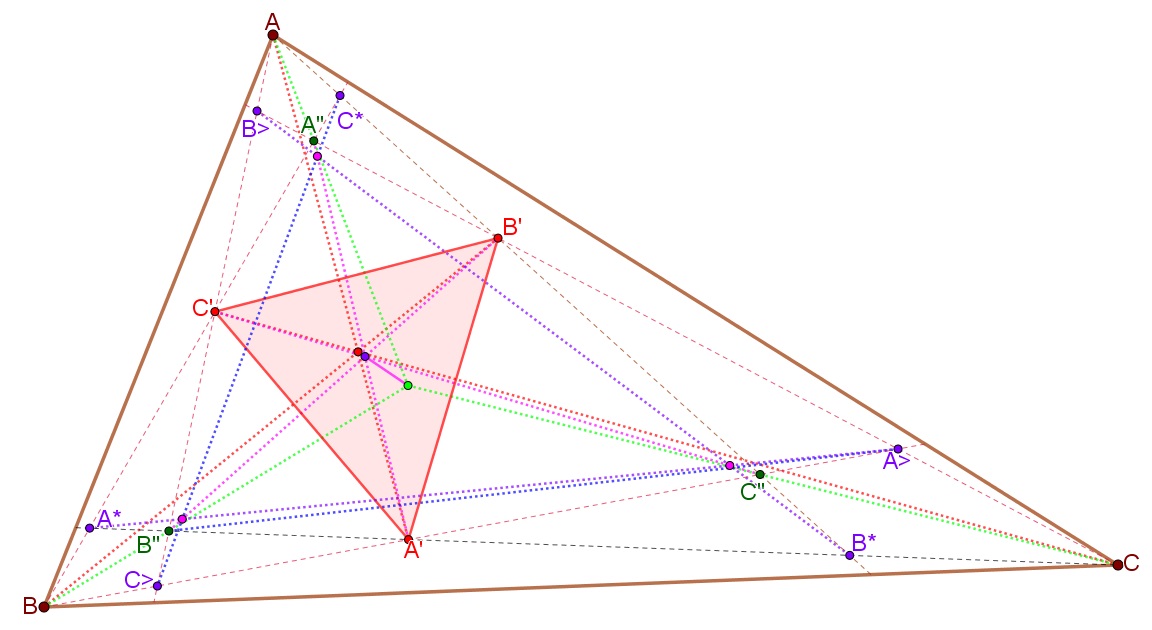
a. Several of the above statements have been shown using various methods. But perhaps all have uniform elementary proofs valid not only for trisectors but also for isogonal lines with respect to each angle, as the following figure indicates. Recall that two lines are isogonal with respect to an angle if the bisector of the angle bisects the angle of the two lines.

b. Are there more concurrencies or colinearities determined by intersections of trisectors of a triangle? Are these also for isogonal lines?