How to formalize this isotopy?

Question

I'm studying the H-Cobordism theorem following the Lectures of John Milnor, and in the proof of the Whitney trick for cancel pairs of self-intersection points I have the next problem with an isotopy which is used without formal definition:

Considering the next plane model composed of two curves $C_0$ and $C'_0$ wich intersect transversally at two points $a$ and $b$ and enclose a disk $D$ in a domain $U$ of $\mathbb{R}^2$.

Milnor says the following:

What is the best way to formalize this isotopy?

Answer 1

I will convert my comment to an answer to this question to take it out of the unanswered queue.

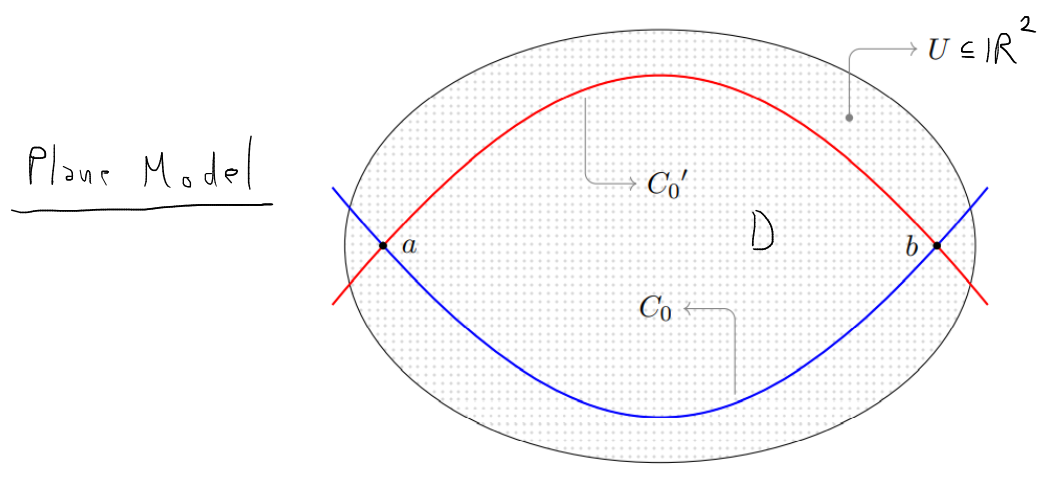
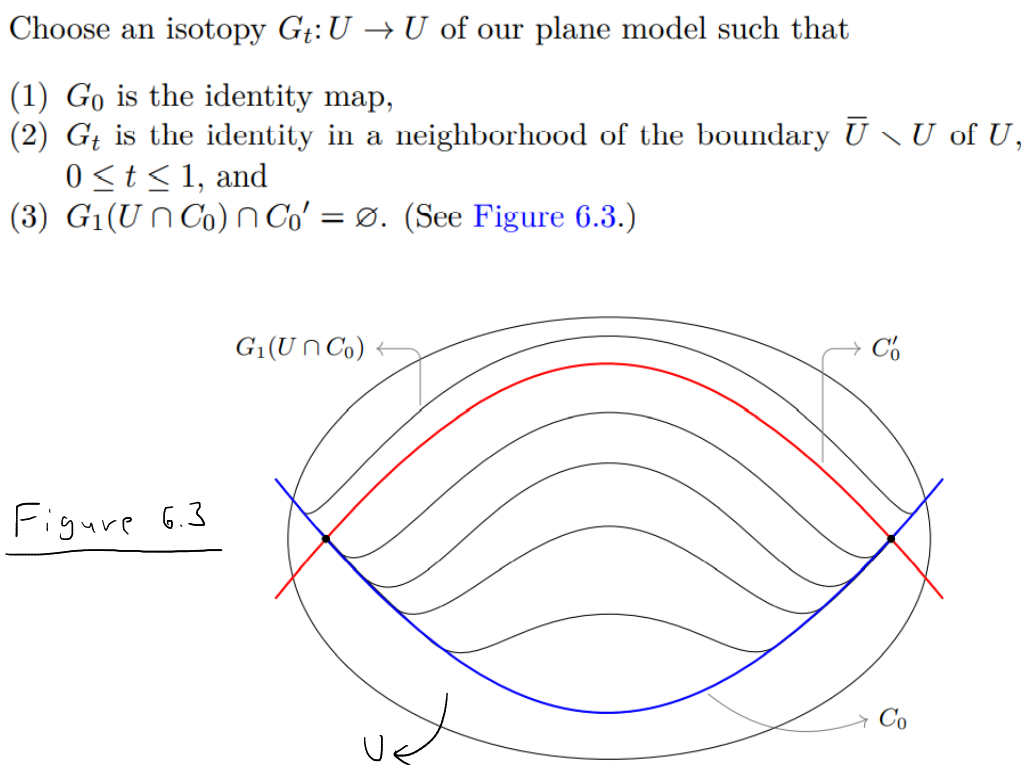
Often a straightforward way to construct isotopies is as the flow of an appropriate vector field. In this planar model, write $C$ for the greatest vertical distance from the blue curve to the red curve, and choose a smooth function $f: \overline U \to [0,2C]$ which is equal to $2C$ on the region enclosed by the blue and red curves, and equal to zero on a neighborhood of $\partial \overline U$. Set $X = f \frac{d}{dy}$ and let $G_t = \Phi_t^X$ be the time-$t$ flow of the vector field $X$.

Then for $t > 1/2$ we have $G_t(U \cap C_0) \cap C_0' = \varnothing$, as the flow on the region enclosed by the two curves is simply flowing upwards at speed $2C$; any point on the blue curve reaches the corresponding point on the red curve within $t \le 1/2$. After leaving the region the flow still moves points directly upwards, but at a slower speed. Condition (3) follows.

Condition (1) follows because the time-$0$ flow of any vector field is the identity, and condition (2) follows because the vector field vanishes in a neighborhood of the boundary.