0
$\begingroup$

This question is a cross-post from math.stackexchange.com. I am reposting it here since I didn't receive an answer there. The original post can be found by this link.

In the following excerpt from Meyer's Probability and Potentials he claims that a non-random process $X(t, \omega) = f ( t )$ is separable for any choice of $f$. And this example seems to work under the definition he gives above.

Paul A. Meyer, Probability and Potentials

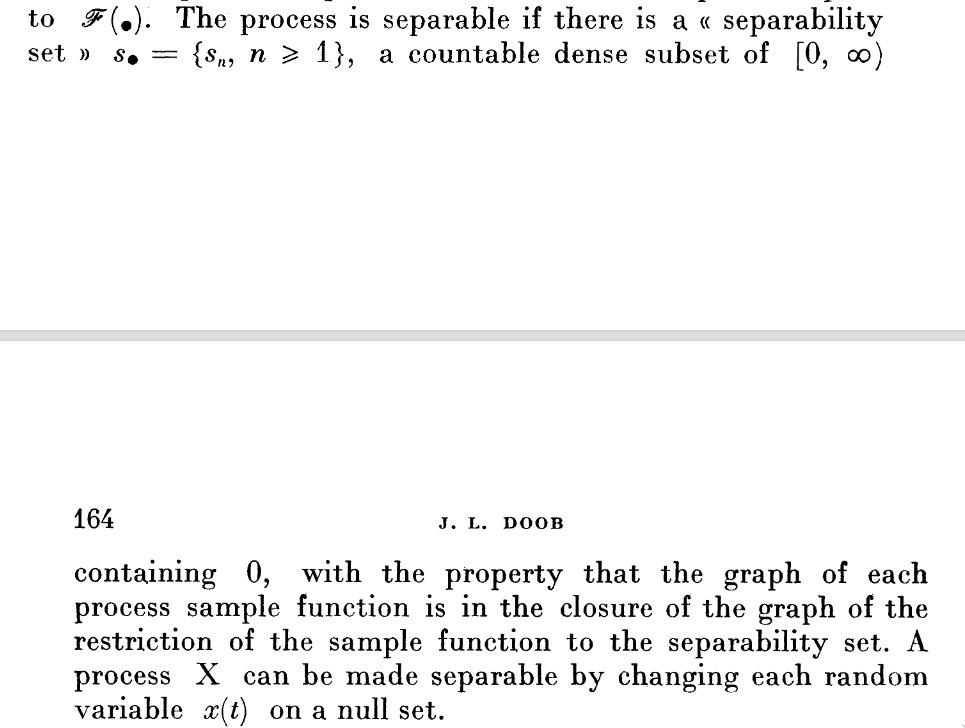
I cannot see, however, how this relates to other definitions of separability, especially the Doob's intuitive definition (see second attachment) as processes closable from a countable set.

J. L. Doob, Stochastic process measurability conditions

Shouldn't we at least ask for some regularity of $f$ in this example? Or even just measurability?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
2
$\begingroup$

"I cannot see, however, how this relates to other definitions of separability, especially the Doob's intuitive definition"

It is clear that, if a stochastic process is separable in the Doob sense, then it is separable in the Meyer sense. (See formulas (13.1), (13.2), and (12.1) in Meyer's book).

Meyer's example cited in your post shows that vice versa is not true: there is a stochastic process separable in the Meyer sense but not in the Doob sense.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Thank you for the answer! So the two definitions do contradict each other, the Meyer's one being less restrictive? $\endgroup$
    – tsnao
    Commented Dec 26, 2022 at 16:14
  • 1
    $\begingroup$ @tsnao : I would not say that "the two definitions do contradict each other" -- that would mean that either one of them excludes the other one. However, Doob's condition (say (D)) never excludes Meyer's conditions (say (M)), and (M) does not always exclude (D). Yet, it is true that (M) is less restrictive than (D). Actually, (M) is less restrictive in two ways: (i) in contrast with (M), (D) specifies the means of identification of the process as the graph closure and (ii) (D) identifies the process pathwise, whereas (M) is only concerned with probabilities. $\endgroup$
    – Iosif Pinelis
    Commented Dec 26, 2022 at 16:30

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .