3
$\begingroup$

It is known that mean curvature flow (MCF) can be used to smoothen out Lipschitz initial data. See Ecker and Huisken, Mean curvature evolution of entire graphsm Ann. Math. (2) 130 (1989), No. 3, 453-471

Can MCF be defined if the initial data is weaker than Lipschitz, say, Holder?

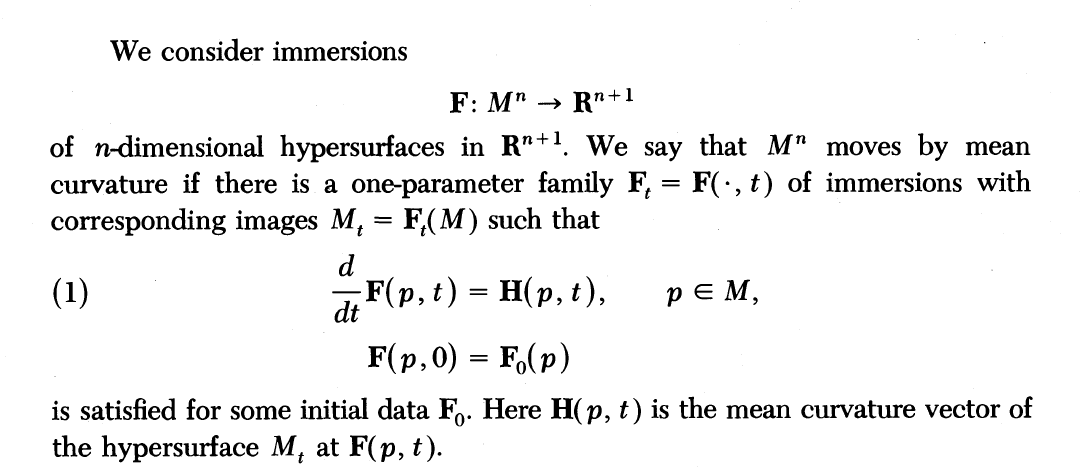
Just for convenience, here I quote the definition of MCF directly from Ecker-Huisken's paper.

enter image description here

Improve this question
$\endgroup$
3
  • $\begingroup$ Admittedly I'm not an expert, but here is how I understand it. Basically it depends on how restrictive you are when defining what you mean by 'MCF'. The least restrictive definition is the so-called 'level set flow'. You can evolve basically any closed set in this flow, but it comes with its own problems, 'fattening' after singular times being the central one. Whether this 'fattening' would happen immediately if one started from, say, the graph of a Weierstrass function... I do not know. [...] $\endgroup$
    – Leo Moos
    Commented Oct 11, 2022 at 10:32
  • $\begingroup$ [...] After re-reading your question, I realised you might be interested in the 'most restrictive' reading of it, namely a family of submanifolds $(M_t)$ which evolve via MCF for all $t > 0$ and have $M_t \to \operatorname{graph} u$ as $t \to 0$, where $u$ is only Holder regular. I don't know whether this is OK. (Unfortunately this seems to have become the central theme of both my comments...) $\endgroup$
    – Leo Moos
    Commented Oct 11, 2022 at 10:38
  • $\begingroup$ @LeoMoos Yes, I'm interested in the situation as you described in the second comment. Also, I'm also interested whether MCF (the 'most restrictive') can be defined just for a short period of time. $\endgroup$
    – Shijie Gu
    Commented Oct 12, 2022 at 4:29

0

Reset to default

You must log in to answer this question.

Browse other questions tagged .