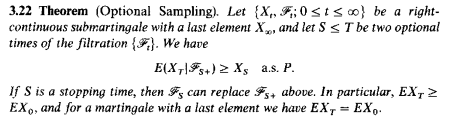$(X_k)_{k \in \mathbb{N}}$ is a sub-martingale, $R_1,R_2$ two stopping times such that $R_1 \leq R_2$.
The optional stopping theorem which I know is the following (from probability theory, independence, interchangeability, martingales: theorem 5 page 248, other reference Shiryaev probability 2: theorem 1 page 119):
Theorem $1$ : letting $X_{R_1}(w)=X_{R_1(w)}(w)1_{\{R_1<\infty\}}(w),$ if $X_{R_1},X_{R_2} \in L^1,$ $\liminf_k\int_{\{R_2>k\}}|X_k|dP=0$ then $E[X_{R_2}|\mathcal{F}_{R_1}] \geq X_{R_1}.$
Consider the following theorem from Shreve-Karatzas book:
In the proof of theorem 3.22, he refers to theorem $9.3.5$ from a course in probability theory:
He refers to exercise $11$, which can be proved easily using theorem $1,$ as a counterexample to theorem $9.3.5$ (not true for all cases):
What's the difference between theorem $1$ and theorem $9.3.5$ ? In theorem $3.22,$ is it necessary to define $X_{\infty}$ as the almost sure limit ? What are the weakest conditions to obtain $E[X_{R_2}|\mathcal{F}_{R_1}] \geq X_{R_1}$ ?