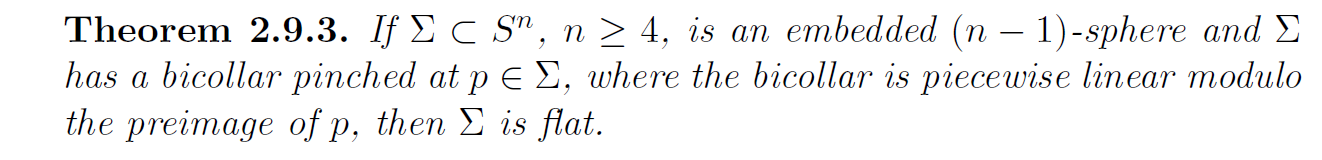Wild spheres in higher dimensions

Question

I was wondering whether anyone had any intuition (or references) as to particular reasons why there are no wild spheres of codimension 1 (where by wild we mean not flat) in higher dimensions $n>3$ that are wild at one point (i.e. locally flat everywhere but a point).

Of course there are the famous examples by Fox and Artin in $n=3$ that have a finite number of wild points and the papers by Cantarelli and Edwards that show that wild simple closed curves in dimensions greater than $3$ contain a Cantor set of wild points, but I am interested if anyone has a reasonable idea as to why $\dim=3$ is so special.

Also I do not know much about wild spheres in codimension other than 1 so I would be interested to find out whether an analogous statement for spheres of arbitrary codimension holds (i.e. if $\Sigma \subset S^n$ is a sphere of codimension $n-k$ that is wild in one point then does it hold that for $n>3$ $\Sigma$ is flat?)

The case of a finite number of wild points is also interesting.

Answer 1

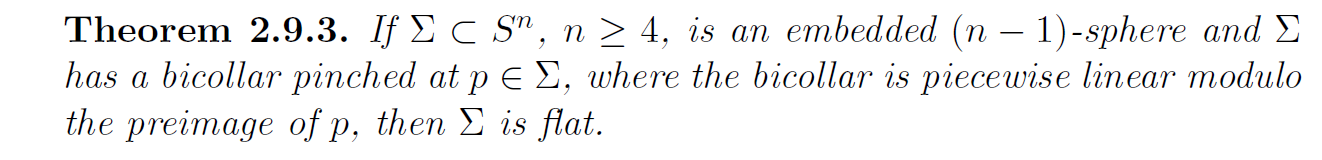
This is an attempt to answer the poster's questions. The initial question is about a result obtained by Cantrell in his doctoral dissertation. Here I quote the version presented in Daverman-Venema's book. 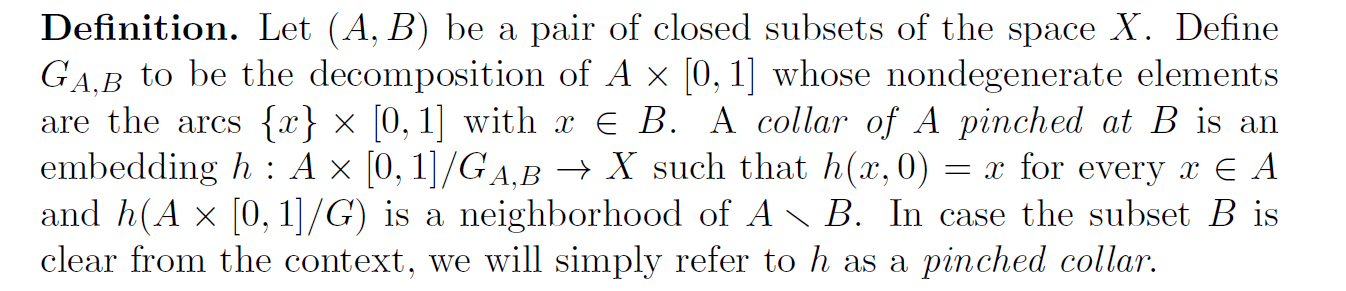The heart of the proof is "Mazur swindle" which Mazur used to prove the generalized Schonflies theorem. The exposition of the proof in Daverman-Venema's book is well written.

Just as the poster observed the distinction between the cases $n=3$ and $n>3$ is interesting. Contrast with $n=3$ the theorem above is not true. There are well-known examples such as the classical Fox-Artin examples Ann. of Math. (2) 49 (1948), 979–990. MR0027512. An alternative resource is Daverman-Venema's book.

Since flatness and wildness are local properties, one may tempt to readily extend Theorem 2.9.3 to imply that codimension one manifolds can have no isolated wild point. However, the extension is less trivial. Because Cantrell's argument depends strongly on the flatness of $\Sigma -p$. Using engulfing techniques ($n\geq 5$), Cernavskii initially obtained this extension, and later Kirby and Cernavskii both obtained an alternative approach, which works in all dimensions.

Theorem. If $B_1$ and $B_2$ are flat $(n-1)$-cells in $\mathbb{R}^n$ such that $B_1\cap B_2 = \partial B_1 \cap \partial B_2$ is an $(n-2)$-cell that is flat in both boundaries, then $B_1 \cup B_2$ is flat.

For Cernavskii's proof, see On singular points of topological imbeddings of manifolds and the union of locally flat cells. (Russian) Dokl. Akad. Nauk SSSR 167 1966 528–530. MR0198447

For Kirby's proof, see The union of flat (n−1)-balls is flat in $R^n$. Bull. Amer. Math. Soc. 74 (1968), 614–617. MR0225328

For more general version of the theorem, see Lemma 7.6.8 and Corollary 7.6.10 on P. 380 in Daverman-Venema's book.

The following two corollaries due to Cernavskii and Kirby, which (almost) immediately follows, are the extensions of Theorem 2.9.3.

Corollary. If $\Sigma$ is an $(n-1)$-sphere in $\mathbb{R}^n$ $(n\geq 4)$ and $W^\ast$ is the set of points of which $\Sigma$ is wildly embedded, then $W^\ast$ contains no isolated point.

Corollary. If $\Sigma$ is an $(n-1)$-manifold in the interior of an $n$-manifold $(n\geq 4)$ and $W^\ast$ is the set of points at which $\Sigma$ fails to be locally flat, then $W^\ast$ is empty or uncountable.

The reason I said "almost" is because to prove the first corollary above, Kirby utilized a result of Lacher (Locally flat strings and half-strings. Proc. Amer. Math. Soc. 18 (1967), 299–304. MR0212805), which is essentially Cantrell's theorem.

As Danny Ruberman pointed out, one may consult Kirby's another paper On the set of non-locally flat points of a submanifold of codimension one. Ann. of Math. (2) 88 (1968), 281–290, where he sharpened the description of the wild set.

Theorem. Suppose $\Sigma$ is an $(n-1)$-manifold in an $n$-manifold $N$ $(n\geq 4)$ such that $\Sigma$ is locally flat modulo a Cantor set $X \subset \Sigma$, where $X$ is tame both as a subset of $\Sigma$ and as a subset of $N$ (a.k.a. twice-flat). Then $\Sigma$ is locally flat.

Again, one may check Daverman-Venema's book (Cor. 7.9.5, P. 404).

Now, why is 3-dimensional case so special? One may consider a special case presented in Daverman-Venema's book (P. 92). The proof relies on the fact (also in Daverman-Venema's book) which may address the poster's question is

For broader interests of this type of the question, as I made in the comments, one may consider engulfing techniques. Heuristically one can expand an open subset of a manifold to engulf a subpolyhedron $P$, provided certain dimension, connectivity and finiteness conditions are satisfied. Among those the codimension $P$ is at least 3. The full power of engulfing techniques require the limitation $n\geq 5$ because then there is room to control the top dimensional part of the space. Look ahead to Chapter 3 in Daverman-Venema's book we may find a lot of nice results obtained using engulfing and see why the dimension restriction matters.

For the poster's second question, the answer would be negative. The counterexample can be found in Daverman-Venema's book.

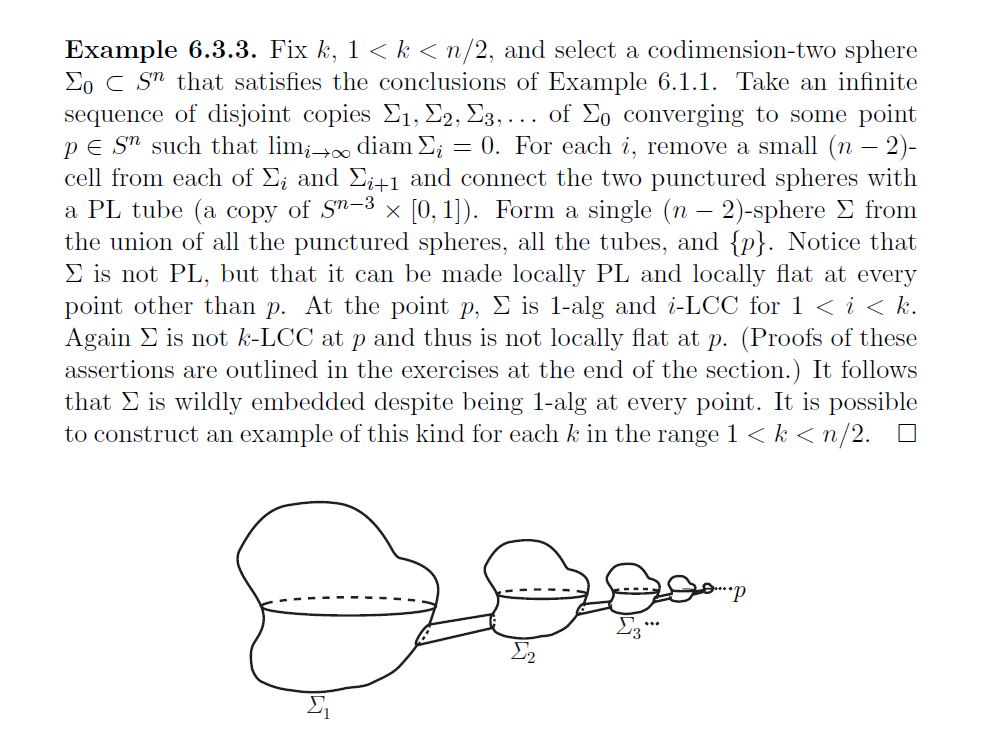
For codimension-two embeddings, an appropriate notion for the wildness is called "knotting". One can define that both globally and locally. The entire Chapter 6 of Daverman-Venema's book is dedicated to unknotting and flatterning.