$\newcommand{\ep}{\varepsilon}\newcommand{\R}{\mathbb R}$First, some simple preliminary cleaning: In the trivial case when $S=\emptyset$, we have $S\infty=\emptyset$. So, in what follows assume that $S\ne\emptyset$. Note that $0=\lim\limits_k\frac1k\,y\in S\infty$, where $y$ is any point in $S$.
For each natural $m$, consider the radial projection
$$A_m:=\Big\{\frac y{|y|}\colon y\in S,|y|\ge m\Big\}$$
on the unit sphere in $\mathbb R^n$ of the part of the set $S$ at distance $\ge m$ from the origin, where $|y|$ is Euclidean norm of $y$. Let then
$$A:=\{0\}\cup\bigcap_m\,\overline{A_m},$$
where $\overline{A_m}$ is the closure of $A_m$.
Then the asymptotic cone $S\infty$ of $S$ coincides with
\begin{equation}
[0,\infty)A=\{tx\colon t\in[0,\infty),x\in A\}.
\end{equation}
This characterization of the asymptotic cone may be easier to visualize.
Proof of this characterization:
(i) Take any $y\in S\infty$, so that $\ep_k y_k\to y$ and $\ep_k\to0$ (as $k\to\infty$) for some sequence $((y_k,\ep_k))$ in $S\times[0,\infty)$. We want to show that then $y\in[0,\infty)A$. If $y=0$, then $y\in A$ and hence $y\in[0,\infty)A$. So, without loss of generality (wlog) $y\ne0$ and hence $|y_k|\to\infty$. So, for each natural $m$ and all large enough $k$ (depending on $m$) we have $z_k:=y_k/|y_k|\in A_m$ and $\ep_k>0$, whence
\begin{equation}
z_k=\frac{\ep_k y_k}{\ep_k|y_k|}\to\frac y{|y|},
\end{equation}
so that $\frac y{|y|}\in\overline{A_m}$. It follows that $\frac y{|y|}\in A$ and thus $y\in[0,\infty)A$, as desired.
(ii) Take any $y\in[0,\infty)A$. We want to show that then $y\in S\infty$. If $y=0$, then $y\in S\infty$. So, wlog $y\ne0$ and hence $\frac y{|y|}\in\bigcap_m\,\overline{A_m}$, so that
for each natural $m$ there is some $x_m\in A_m$ such that $|\frac y{|y|}-x_m|<\frac1m$. Since $x_m=\frac{y_m}{|y_m|}$ for some $y_m\in S$ with $|y_m|\ge m$, for all natural $m$ we have
\begin{equation}
|y-\ep_m y_m|<\frac{|y|}m,
\end{equation}
where $\ep_m:=\frac{|y|}{|y_m|}\to0$ (as $m\to\infty$). Thus, $y\in S\infty$, as desired.
In your comment, you also seem to ask how to use this characterization of the asymptotic cone to show that for $S=\{(a,b)\in\R^2\colon b>a^2\}$ we have $S\infty=C:=\{(0,b)\in\R^2\colon b\ge0\}$.
This follows because here $\overline{A_m}$ is the set of all unit vectors in $\R^2$ with a positive second coordinate and the absolute value of the first coordinate
$\le\sqrt{\dfrac{\sqrt{4 m^2+1}-1}{2m^2}}\to0$ (as $m\to\infty$).
Here is the set $\overline{A_m}$ for $m=10$ (red):

Comment: The visualization suggestion in the first comment by YCor should apparently work when the set $S$ is convex, but not in general. Pictured below is the set $S\cap[-100,100]^2$ (blue) with
$$S:=\{e^{t/5}(r\cos t,r\sin t)\colon 1\le r\le2,t\in\R\},$$
a "fat logarithmic spiral" set:
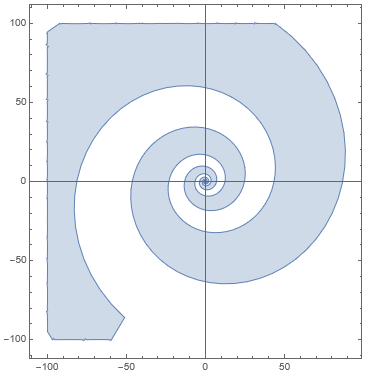
Here the set $S\cap[-m,m]^2$ will not look like a cone, for any natural $m$, because the image of $S$ under any homothety with the fixed point at the origin is obtained by a rotation of $S$.


