 (source: Wayback Machine)(source)
(source: Wayback Machine)(source)
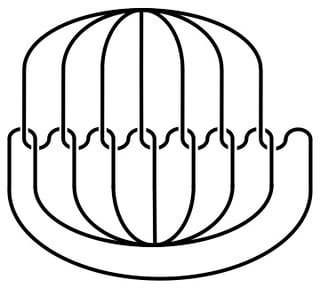
The exterior of Suzuki's Brunnian graph on $n$-edges, here pictured with $n=7$, is irreducible, atoroidal, boundary incompressible, and acylindrical. See
Luisa Paoluzzi and Bruno Zimmermann. On a class of hyperbolic 3-manifolds and groups with one defining relation. Geom. Dedicata, 60(2):113–123, 1996
or
Akira Ushijima. The canonical decompositions of some family of compact orientable hyperbolic 3-manifolds with totally geodesic boundary. Geom. Dedicata, 78(1):21–47, 1999.
(I think these manifolds may be contained in Bruno's list also.)
