For $n=5$, this has been shown by Eppstein:
D. Eppstein, Happy endings for flip graphs, Journal of Computational Geometry 1 (2010), no. 1, 3--28.D. Eppstein, Happy endings for flip graphs, Journal of Computational Geometry 1 (2010), no. 1, 3--28.
For odd $n>5$, one could consider the polygon bounded by the longest diagonals.
It may be defined as the intersection of the half-planes containing $(n+1)/2$ vertices of $P$.
For $n=9$, this intersection may be empty (for example, if the nine vertices form three triples and each of the triples is placed very close to a vertex of a regular triangle).
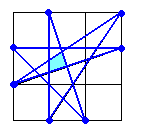
For $n=7$, this intersection is non-empty. However, it may be free of lattice points:
take, for example, the polygon $P$ with vertices
$[0,1],
[1,0],
[2,0],
[3,2],
[3,3],
[1,3],
[0,2]$.