Suppose every prime $n$ could "explode" once.
An explosion results in $\lfloor \alpha \ln n \rfloor$ particles being
uniformly distributed over the integers in a range $n \pm \lfloor \beta \ln n \rfloor$.
If a particle hits a composite or a previously exploded prime, nothing happens.
If a particle hits a new prime $p$, then $p$ explodes under the same rules.
Here is a little simulation starting at $n=23$,
with $\alpha=10$ and $\beta=5$:
Animation http://cs.smith.edu/~orourke/MathOverflow/ExplodingPrimes1.gif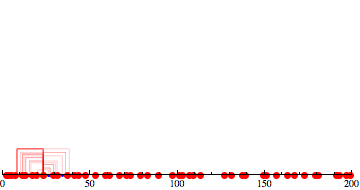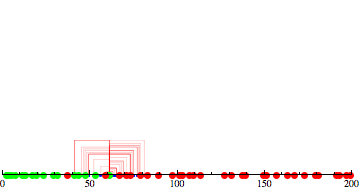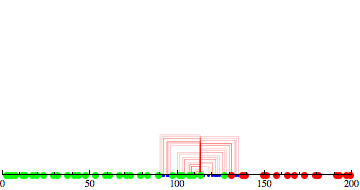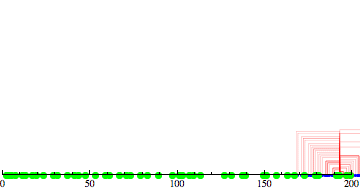
So, on the first step, $n=23$ explodes into $\lfloor 10 \ln 23 \rfloor = 31$
particles, uniformly spreading over $23 \pm 15$. In the run depicted, these particles hit four primes:$11, 13, 29, 31$. Then each of those explodes; and so on. In the last frame, $199$ is hit.
Q1. For which $\alpha$ and $\beta$, if any, will this process almost surely explode an infinite number of primes?
I am hoping the answer is independent of the starting $n$.
Being largely ignorant of number theory, I am wondering if current knowledge of the distribution of the primes is sufficient to answer this question. Thanks for insights or even speculations!
Q1 Answered. quid shows that a ballistic range of $\pm \beta \log n$ is not enough to bridge the prime gaps: $\pm \beta \log^2 n$ conjecturally suffices, but a range of more than $\pm \beta \sqrt{n}$ is all that current technology can prove.
Addendum. Both quid and joro confirm that even assuming RH (the Riemann Hypothesis), the range needed for my simulation to provably explode all primes is at least $\pm \beta \sqrt n \log n$. May I add this question:
Q2. What is the minimum ballistic $\pm$-range that would suffice to provably (under "current technology") explode (with sufficent $\alpha$) all primes without any RH or otherwise conjectural assumptions?
