Sorry, this is an answer to an other question. (I did not read the question carefully.)
Question: For which $k$, $k$ squares can tile the surface of cube.
Answer: $k=6\cdot(n^2+m^2)$.
Here is a tiling with $k=30$, $n=1$ and $m=2$.
 $k=30$." />
$k=30$." />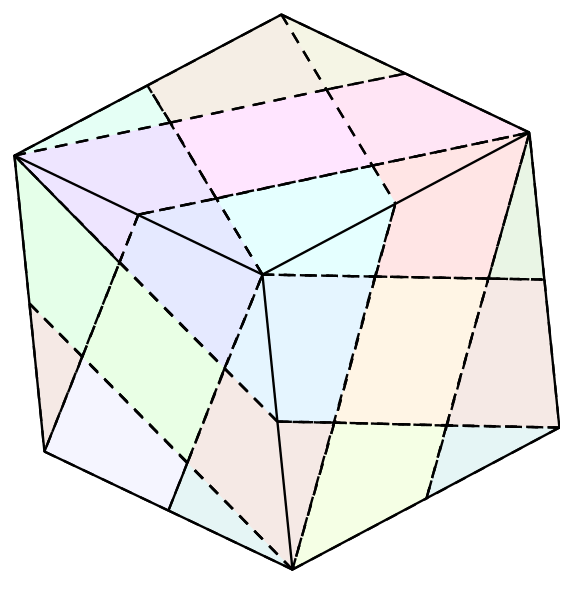
(source: psu.edu)
It is obvious if the tiling is vertex-to-vertex.
If the tiling is not vertex-to-vertex, you get a closed geodesic formed by overlaping sides. Then you can shift squares on one side of the geodesic to make the tiling "more vertex-to-vertex". Repeating this operation you can make the tiling to be vertex-to-vertex.

