C.N. Little listing the Perko pairPerko pair as different knots in 1885 (10161 and 10162). The mistake was found almost a century later, in 1974, by Ken Perko, a NY lawyer (!)
For almost a century, when everyone thought they were different knots, people tried their best to find knot invariants to distinguish them, but of course they failed. But the effort was a major motivation to research covering linkage etc., and was surely tremendously fruitful for knot theory.
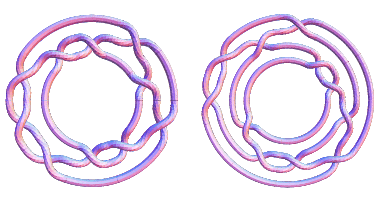 (source)
(source)
Update (2013):
This morning I received a letter from Ken Perko himself, revealing the true history of the Perko pair, which is so much more interesting! Perko writes:
The duplicate knot in tables compiled by Tait-Little [3], Conway [1], and Rolfsen-Bailey-Roth [4], is not just a bookkeeping error. It is a counterexample to an 1899 "Theorem" of C.N. Little (Yale PhD, 1885), accepted as true by P.G. Tait [3], and incorporated by Dehn and Heegaard in their important survey article on "Analysis situs" in the German Encyclopedia of Mathematics [2].
Little's `Theorem' was that any two reduced diagrams of the same knot possess the same writhe (number of overcrossings minus number of undercrossings). The Perko pair have different writhes, and so Little's "Theorem", if true, would prove them to be distinct!
Perko continues:
Yet still, after 40 years, learned scholars do not speak of Little's false theorem, describing instead its decapitated remnants as a Tait ConjectureTait Conjecture- and indeed, one subsequently proved correct by Kauffman, Murasugi, and Thistlethwaite.
I had no idea! Perko concludes (boldface is my own):
I think they are missing a valuable point. History instructs by reminding the reader not merely of past triumphs, but of terrible mistakes as well.
And the final nail in the coffin is that the image above isn't of the Perko pair!!! It's the `Weisstein pair' $10_{161}$ and mirror $10_{163}$, described by Perko as "those magenta colored, almost matching non-twins that add beauty and confusion to the Perko Pair page of Wolfram Web’s Math World website. In a way, it’s an honor to have my name attached to such a well-crafted likeness of a couple of Bhuddist prayer wheels, but it certainly must be treated with the caution that its color suggests by anyone seriously interested in mathematics."
The real Perko pair is this:

You can read more about this fascinating story at Richard Elwes's blogRichard Elwes's blog.
Well, I'll be jiggered! The most interesting mathematics mistake that I know turns out to be more interesting than I had ever imagined!
1. J.H. Conway, An enumeration of knots and links, and some of their algebraic properties, Proc. Conf. Oxford, 1967, p. 329-358 (Pergamon Press, 1970). 2. M. Dehn and P. Heegaard, Enzyk. der Math. Wiss. III AB 3 (1907), p. 212: "Die algebraische Zahl der Ueberkreuzungen ist fuer die reduzierte Form jedes Knotens bestimmt." 3. C.N. Little, Non-alternating +/- knots, Trans. Roy. Soc. Edinburgh 39 (1900), page 774 and plate III. This paper describes itself at p. 771 as "Communicated by Prof. Tait." 4. D. Rolfsen, Knots and links (Publish or Perish, 1976).
