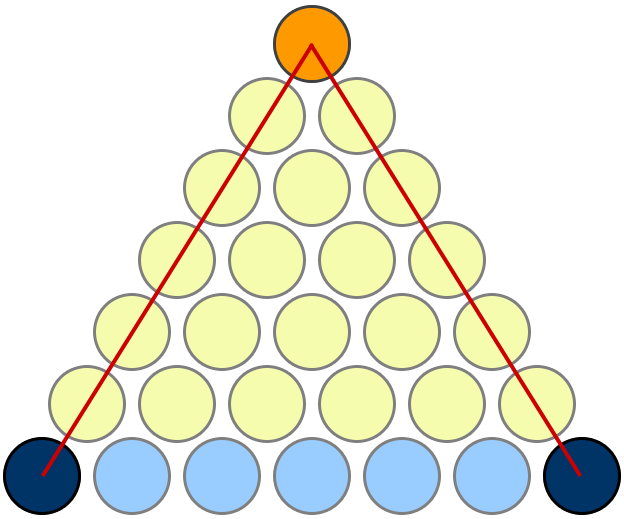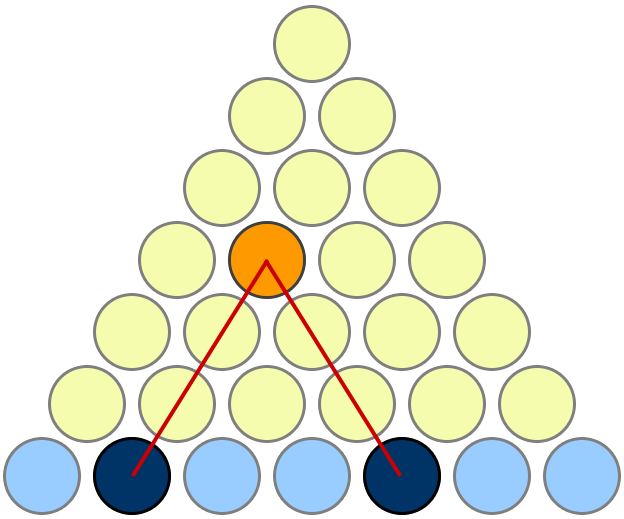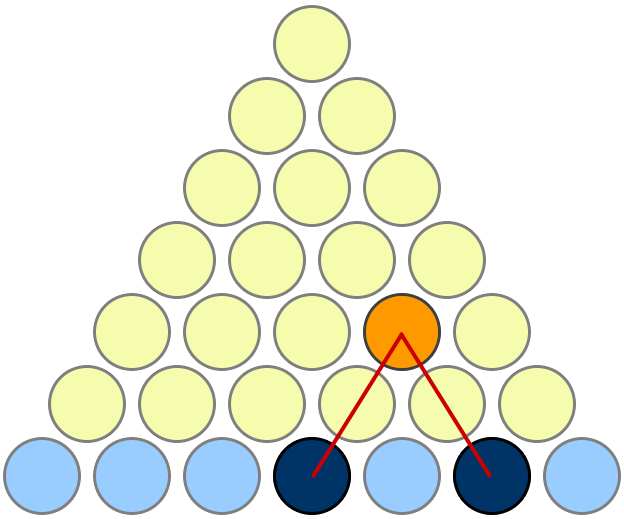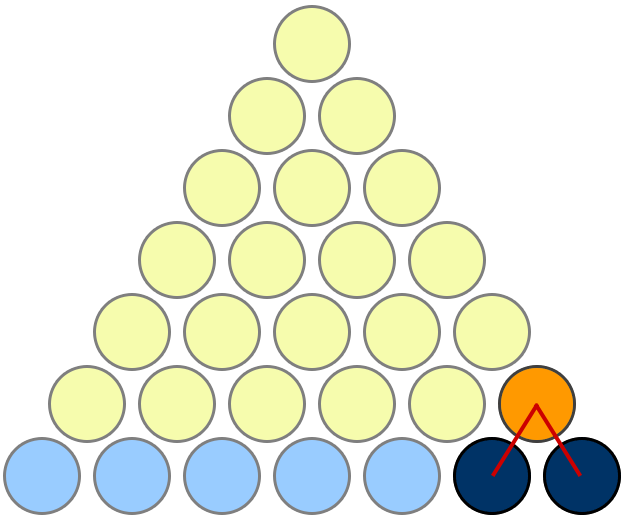
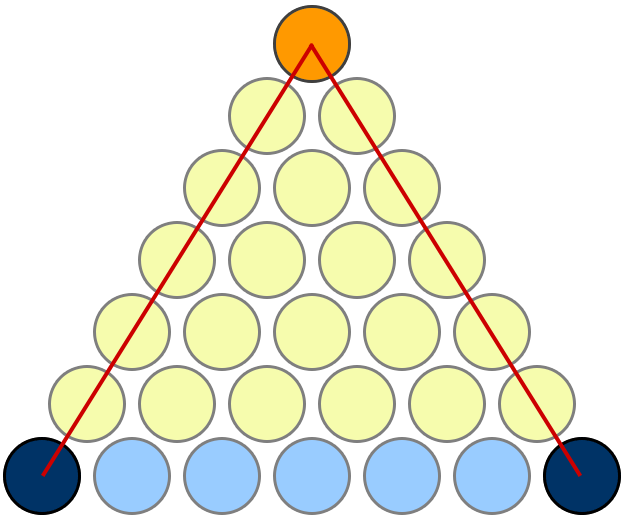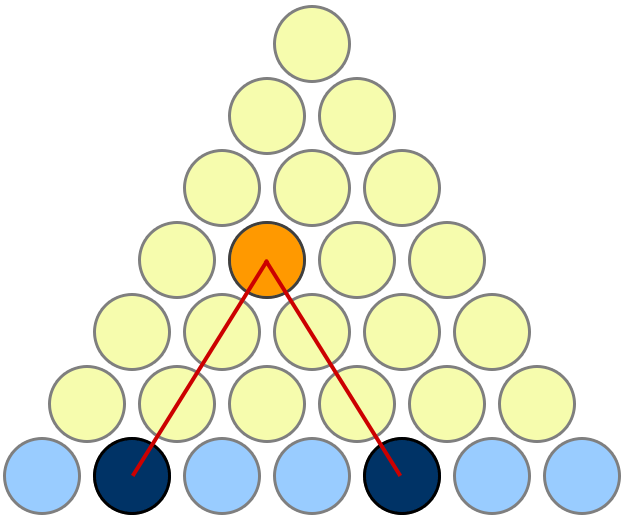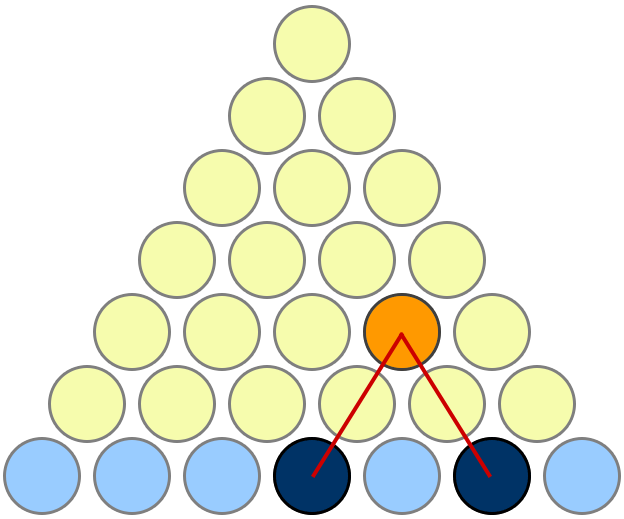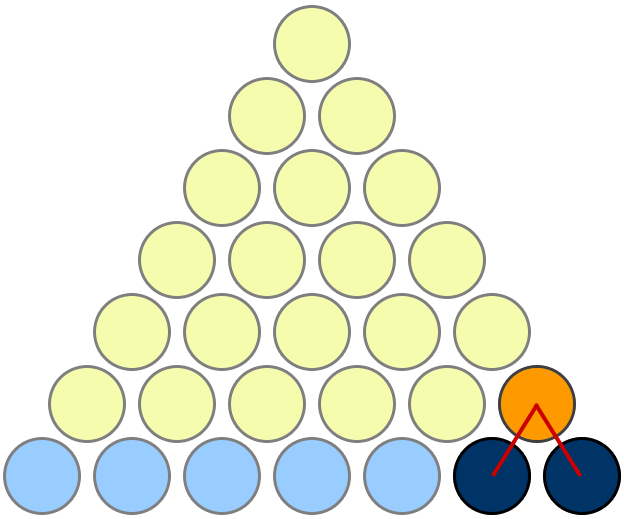
A proof of the identity $$1+2+\cdots + (n-1) = \binom{n}{2}$$
(Adapted from an entry I saw at Wolfram Demonstrations, see also the original faster animation)
This proof was discovered by Loren Larson, professor emeritus at St. Olaf College. He included it along with a number of other, more standard, proofs, in "A Discrete Look at 1+2+...+n," published in 1985 in The College Mathematics Journal (vol. 16, no. 5, pp. 369-382, DOI: 10.1080/07468342.1985.11972910, JSTOR).