Let $a(t)$ and $b(t)$ be two smooth, nested convex curves in the plane, $t\in[0,1]$:
Nested Curves http://cs.smith.edu/%7Eorourke/MathOverflow/NestedCurves.jpg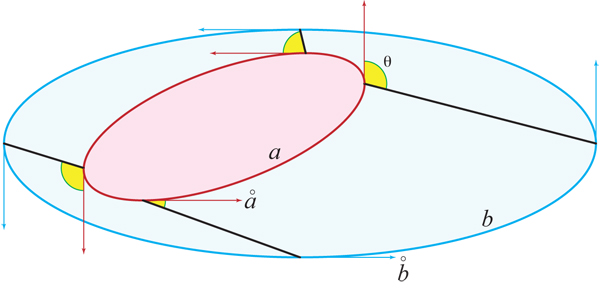
Suppose the parametrization of $a()$ and $b()$ is such that $\dot{a}(t)$ is parallel to $\dot{b}(t)$:
at time $t$, the tangents at $a(t)$ and $b(t)$ are parallel. Let $ab(t) = b(t)-a(t)$ be the vector
from $a(t)$ to $b(t)$, and let $\theta(t)$ be the counterclockwise angle from $ab(t)$ to $\dot{a}(t)$.
I would like to claim that
$\theta(t) = \pi/2$ at least twice within $t\in[0,1]$.
My proof of this is inelegant, relying on the length $|ab(t)|$, essentially showing that if, e.g, $\theta(t) < \pi/2$ for all $t$, then $|ab(1)| >|ab(0)|$ (contradicting $|ab(1)| = |ab(0)|$). But I feel there might be a clever way to achieve this via the mean-value theorem that I am not seeing. Also, perhaps "twice" can be replaced by "four times," and perhaps "nested" need not be assumed. So I am seeking a cleaner proof that may yield further insights. Thanks for contributing ideas or pointing me in the right direction!
