The Koebe–Andreev–Thurston theorem states that any planar graph can be represented
"in such a way that its vertices correspond to disjoint disks, which touch if and only if
the corresponding vertices are adjacent" (to quote Günter Ziegler, Lectures on Polytopes, Springer, 1995 p.117.
(See also the Wikipedia article, "Circle packing theorem.")

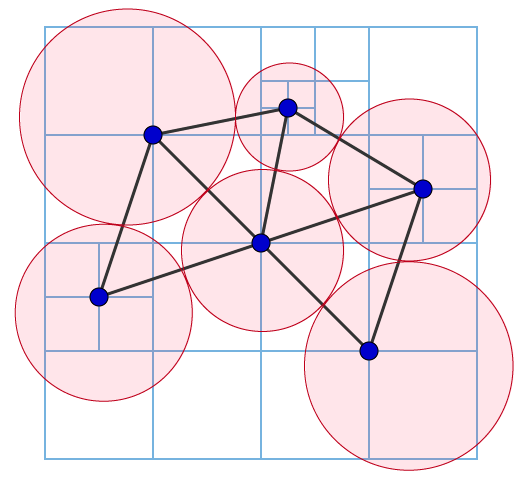
(source: uci.edu)
(Image due to David Eppstein, here.)
What is the corresponding statement for spheres in $\mathbb{R}^3$? Every graph $G$ satisfying property $X$(?) can be represented by touching spheres.
This is surely known—Thanks for pointers!
