I'm not sure if this is what you're looking forhelpful, but here is an example. The following picture shows the filled Julia set for $z^6 - 1$.
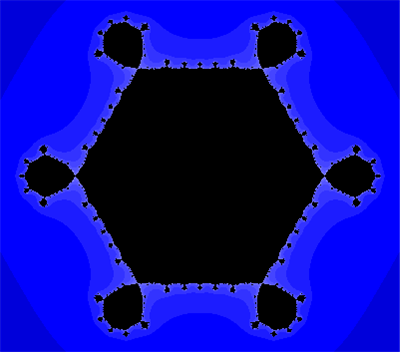
and the following picture shows the filled Julia set for $(z^2-1)^3$:
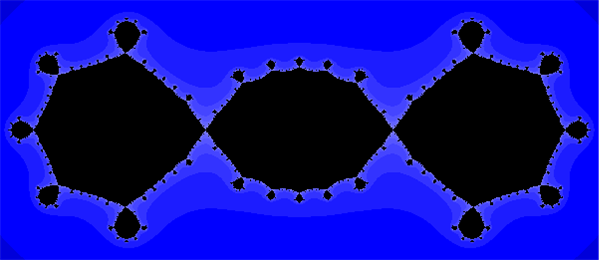
This is the case where $f(z) = z^2 - 1$ and $g(z) = z^3$. Note that the bottom image is a double cover of the top, while the top image is a triple cover of the bottom.
(These images were produced using Mathematica.)
