I asked Jon Lenchner, an expert on point-line incidences, and he told me the question (in dual form) was posed in Grünbaum's 1971 book Arrangements and Spreads, and fully answered in a paper by Peter Salamon and Paul Erdős: "The solution to a problem of Grünbaum," Canad. Math. Bull. 31: 129-138 (1988). Here are its first two sentences:
In the paper below we characterize for large $n$ the possible values of the number of connecting lines determined by a set of $P_n$ points in the plane, where a connecting line is any straight line containing at least two points of $P_n$. This solves a problem posed by Grünbaum [5,6] which asks for the sequence of all integers $m$ with the property that some configuration of $n$ points determine exactly $m$ lines.
([6] is Arrangements and Spreads; [5] is Erdős's earlier partial solution.)
They obtain exact expressions "for the lower end of the continuum of values leading
down from $\binom{n}{2}-4$." "The possible values...can be seen to bear a strong resemblance
to physical spectra."
The lower end of the continuum grows as $n^{3/2}$ (with constant 1).
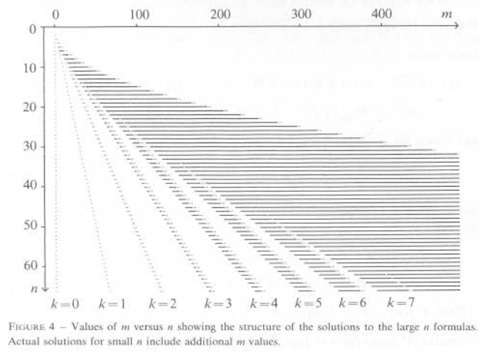
Here are two figures from the paper:
SE Fig1 http://cs.smith.edu/%7Eorourke/MathOverflow/SalamonErdosFig1.jpg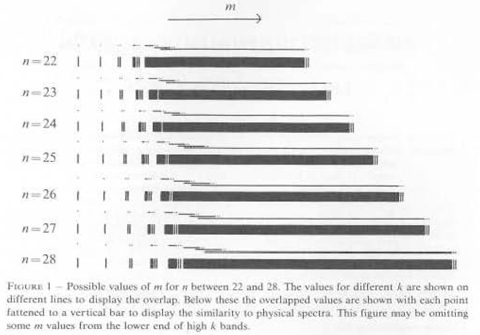
SE Fig4 http://cs.smith.edu/%7Eorourke/MathOverflow/SalamonErdosFig4.jpg