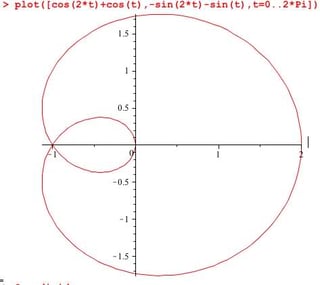
If $a$ satisfies $a=\sqrt{z+a}$ then $a/z = 1/(a-1)$ which usually does not have modulus $1$. So for $1/(a-1) = e^{i\theta}$ we get $z = e^{-2i\theta}+e^{-i\theta}$.
Copied image to imgur.com, as it was not being displayed because of the new https rule.
jeq
- 1.2k
- 5
- 16
- 21