As I said in the comment above, I think that the set of points $t\in[0,1]$ where a square-filling curve with strictly increasing area defines a convex $f([0,t])$, is a nowhere dense closed set containing $0$ and $1$, and conversely, any such set can be obtained this way. While I'm not sure about how to show that such a set is always nowhere dense, the other direction seems easier to pursue, and allows a nice construction (I'll try to include a picture too). Precisely:
For any closed nowhere dense subset $C$ of $I:=[0,1]$ containing $0$ and $1$ there exists a square-filling curve $f:I\to I\times I$ with the property that $f([0,t])$ has area $t$ for all $t\in I$, and $f([0,t])$ is convex exactly for all $t\in C$.
For convenience, I'll describe the construction with a slight variation in the parametrization, requiring that the curve satisfies, for all $t\in C$, $f(t)=(0,t)$ (thus, at any time $t\in C$ it touches the right vertical edge of the square, at heigh $t$). The area will be strictly increasing, for instance with $\operatorname{Area}\big(f([0,t])\big)=\phi(t):=3t^2-2t^3$ for all $t\in I$ (any other homeomorphism $\phi$ of $[0,1]$ in itself such that $\phi(t)=o(t)$ and $\phi(1-t)=o(t)$ as $t\to0$ works as well). Of course, if one started with $C\:':=\phi(C)$, then one finds a curve $f\circ \phi^{-1}$ parametrized in "arc-area", as initially stated.
To start the construction we first need to fix the subsets $f([0,t])$, for all $t\in C$. To this end, note that there exists a nested family of closed, convex subsets of the square, $\{A_t\}_{t\in C}$, such that $A_0:=\{(1,0)\}$, $A_1:=I^2$, $\operatorname{Area}(A_t)=\phi(t)$ for all $t\in C$, and $\operatorname{diam}(A_ s \setminus A_r)=o(1)$ as $|s-r| \to0 $, (uniformly for $r$ and $s$ in $C$).
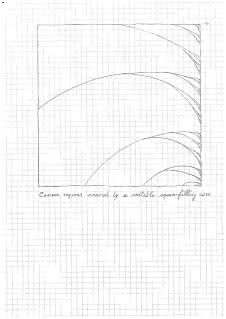
Instead of entering the details of the construction of these $A_t$, let's just say that they can be realized e.g. as sub-graphs of a family of concave functions $\alpha_t:I\to ]-\infty,1]$: $$A_t:=\{(x,y)\in I^2\, :\, \alpha_t(x)\ge y\}$$ where $\alpha _ s\leq\alpha _t$ for $s\leq t$ and $\int_0^1\alpha^{+} _ t(x)dx=\phi(t)\, .$
The graphs of these functions appear as a forest of binary trees leaning their branches towards the right vertical edge, with (possibly uncountable) leaves exactly at the set $\{1\}\times C$. They disconnect the square into a countable family of open regions, one for each component $J$ of $I\setminus C\, .$
The curve $f:I\to I^2$ is defined to be $f(t)=(0,t)$ as said, for all $t\in C$. On any open interval $J:=]r,s[$ which is a component of $I\setminus C$, define $f_{|J}$ to be a Peano-like curve filling the set ${ A_s\setminus A_r }$ up to its closure, with end-points $f(s)=s$ and $f(r)=r$ as said, and parametrized in such a way that $\operatorname{Area}(f[s,t])=\phi(t)-\phi(s)$ for all $t\in J\, .$
This defines a curve $f:I\to I^2$ with the stated properties. Note that the continuity is ensured by the requirement that $\operatorname{diam}(A_t \setminus A_s)=o(1)$ as $|t-s| \to0 $, (uniformly). Since we want the sets $f([0,t])$ to be convex at exactly the points $t\in C$, a small care is needed in order to avoid creating new convex sets $f[0,t]$ for $t\in I\setminus C$, but a small thoughts shows that this is not a problem (for instance the original Peano curve does have this property).
![alt text][1]
[1]: https://i.sstatic.net/Ybm1t.jpg