If you allow the open sets $X$, $U$ and $V$ to be disconnected, you get a counter-example by taking a countable example and changing points to pairwise disjoint balls.
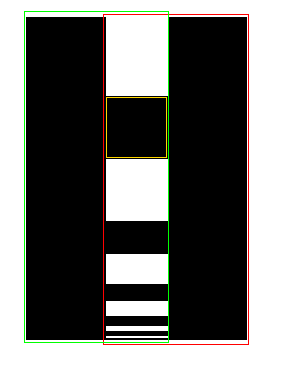
Edit 2: Here is a modified version of Joel's example, which was later deleted. Define $U$ to be the open set inside the green rectangle and $V$ to be the open set inside the red rectangle. Just for notation, suppose that the left-bottom corner is $(0,0)$ and that the width of the "house" is $1$.
Define $f \colon U \to U$ be a mapping $$(x,y) \mapsto \left(\frac12x,y\right)$$ on the part of $U$ which does not include the "chimney", i.e. the top left box. Correspondingly, the part of the mapping $g \colon V \to V$ which maps everything but the chimney is defined as $$(x,y) \mapsto \left(\frac12(x+1),y\right).$$ The chimneys are then mapped with homoemorphisms to the missing parts of $U$ and $V$. Now the set $U \cap V$ is the middle part of the house. The set inside the yellow rectangle has two pre-images and has positive area.
http://users.jyu.fi/~tamaraja/temp/house.gifRemark: This example does not differ that much from the first one which I posted. Essentially we just extend the mappings defined on the balls slightly (and rearrange the balls if this is not possible otherwise) to make the sets $U$ and $V$ connected.