[Edited to correct the final position (which didn't work before: 2 Bh1+! Bg2 3 Rf1 Bb1 etc.), and — while I'm at it — to remove a few superfluous pawns]
Here's yet another mechanism, which also allows arbitrarily long chains of irreversibility. The new construction is suggested by what may be an unintenional feature/bug in the original question:
[...] the game must be extendable at least two moves in both direction from both A and B.
The number seems arbitrary (why two moves rather than three or 23?), and I'm guessing that the intention was to guarantee that one can continue indefinitely forwards or backwards from both positions but give an easily checked criterion. However, it's possible for a position to be extendable $n$ moves and no further for any given $n$, as long as we're allowed an arbitrarily large board and supply of pieces (as the question proposal did explicitly allow). For example: let Position APosition A have White rook a5, bishops c2,e2,g2, and pawns b3,d3,f3,h3 vs. Black bishops b1,d1,f1,h1, plus any immobile setup that traps both kings above the 3rd row:
http://www.janko.at/Retros/d.php?ff=5k2/5P2/5Pp1/R5p1/6Kp/1P1P1P1P/2B1B1B1/1b1b1b1b
(source: janko.at)
and play 1 Ra1 to get Position BPosition B. Not only can't we get back from B to A, but after a few moves (but more than 2) we must reach stalemate: 1...Ba2 2 Bb1 (or 2 Rb1 stalemate, or 2 Rc1 Bc2 stalemate) 2...Bc2 3 Bd1 Be2 4 Bf1 and now 4...Bg2 is stalemate.
For a long irreversibility chain not ended by stalemate, remove the White and Black bishops on g2 and h1, put the White king on h1, and add Black pawns on h2 and g3:
http://www.janko.at/Retros/d.php?ff=5k2/5P2/5P2/R7/8/1P1P1PpP/2B1B2p/1b1b1b1K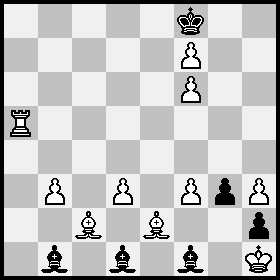
(source: janko.at)
Now after 1 Ra1 play can continue indefinitely, but only with 1...Ba2 2 Bb1 Bc2 3 Bd1 Be2
http://www.janko.at/Retros/d.php?ff=5k2/5P2/5P2/8/8/1P1P1PpP/b1b1b2p/RB1B3K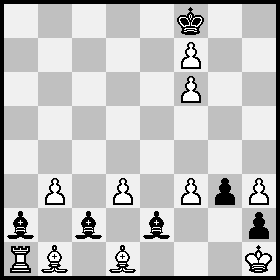
(source: janko.at)
and now 4 Kg2 Bf1+ 5 Kh1 Be2 etc. This extends naturally to wider boards, with a correspondingly longer sequence between 1 Ra1 and the onset of forced repetition.
