The limit shape definitely depends on the contour. Richard Kenyon's "Lectures on dimers" (PDF link) explains the status (p.32):
For general boundary conditions finding the analytic function $Q$ in [equation] (11) which describes the limit shape is difficult. For boundary conditions resembling those in Figure 4, however, one can give an explicit answer.
The above only makes full sense in the context of his notes, but he can compute the
limit shape in the case below, where it is a cardiod:
Kenyon http://people.csail.mit.edu/%7Eorourke/MathOverflow/KenyonLimitShape.jpg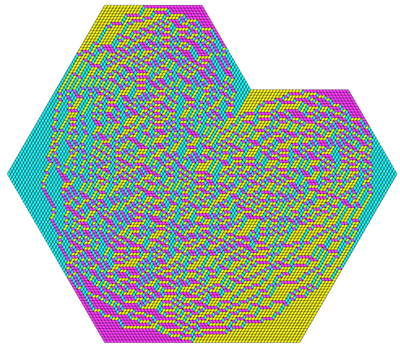
I believe that the limit shape can be computed for all polygonal approximations of a certain
type, those "resembling those in Figure 4."
