I arrived to Penrose's paper Applications of negative dimensional Tensors after reading some bits of Baez's Prehistory (link) and the first two chapters of Turaev's Quantum invariants of knots and 3-manifolds (link). The main result in the last one is a presentation of $\text{Rib}$ (the category of ribbon graphs) by a set of generators and relations (braiding, twist and their inverses, duality morphisms...).
On the other hand Penrose seems to add to the natural braided ribbon structure also a differential one finding generators also for the (anti)symmetrization and covariant-derivative operations. Are there some easily-found references for a structure theorem similar to Lemma 3.1.1 in the book of Turaev?
Edit: I'm sorry I forgot the second question: I have some problems in figuring out the "contraction" in Penrose notation, because in Turaev exposition the juxtaposition of "coupons" seems to be reserved to the composition of morphisms (contraction contrarily implicates some sort of Einstein summation, am I right?)
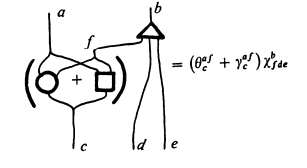
This is the last edit, I promise: the following convention to contract the sum of two tensors alt text http://img853.imageshack.us/img853/6413/screenshot2lw.png
doesn't make any sense to me.. How can the "f" leg of $\chi^b_{fde}$ split into two?