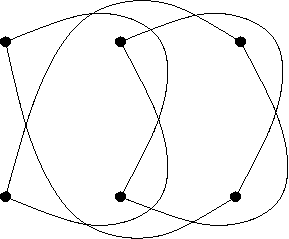
Conway's Thrackle Conjecture: $E \le V$ in any "thrackle," a particular type of drawing of a graph of $V$ vertices and $E$ edges in the plane, requiring that every pair of edges "meet" once. Dangerously addictive! And advances made every few years; it is by no means an isolated conjecture.
Thrackle http://upload.wikimedia.org/wikipedia/en/thumb/4/44/6-cycle_thrackle.png/220px-6-cycle_thrackle.png