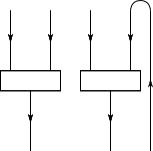
I find that thinking in string diagram pictures is easiest for me. The identification of homs comes from taking a map $X \otimes Y \to Z$ and bending one of the strings around to the other side, as in the picture below.
http://math.uchicago.edu/~ejenkins/misc/hom-tensor.png What we get is a map $X \to Z \otimes Y^{\ast}$. How do you know that this is $Y^{\ast}$ and not ${^{\ast}}Y$? Well, I call $Y^{\ast}$ the left right dual (maybe other people call it the right left dual), and it's the one where the arrow on the string goes from right to left, at least the way I draw the diagrams. The other way to remember it is that the ${\ast}$ goes on the inside in the evaluation pairing (and hence on the outside in the coevaluation).
I don't think people will ever agree on conventions for which way string diagrams go, or which one is the left dual and which one is the right dual, but I can at least be internally consistent with these conventions.